1049:
9329:
9112:
9350:
9318:
9387:
9360:
9340:
5614:
2621:
2982:
7241:
3351:
20:
2788:
2780:
5359:
7020:
2362:
2101:
5082:
5609:{\displaystyle {\begin{aligned}{\mathfrak {N}}_{0}&=\mathbb {Z} /2,\\{\mathfrak {N}}_{1}&=0,\\{\mathfrak {N}}_{2}&=\mathbb {Z} /2,\\{\mathfrak {N}}_{3}&=0,\\{\mathfrak {N}}_{4}&=\mathbb {Z} /2\oplus \mathbb {Z} /2,\\{\mathfrak {N}}_{5}&=\mathbb {Z} /2.\end{aligned}}}
2499:
3841:
In low dimensions, the bordism question is relatively trivial, but the category of cobordism is not. For instance, the disk bounding the circle corresponds to a nullary (0-ary) operation, while the cylinder corresponds to a 1-ary operation and the pair of pants to a binary operation.
7236:{\displaystyle {\begin{aligned}\Omega _{0}^{\text{SO}}&=\mathbb {Z} ,\\\Omega _{1}^{\text{SO}}&=0,\\\Omega _{2}^{\text{SO}}&=0,\\\Omega _{3}^{\text{SO}}&=0,\\\Omega _{4}^{\text{SO}}&=\mathbb {Z} ,\\\Omega _{5}^{\text{SO}}&=\mathbb {Z} _{2}.\end{aligned}}}
7470:
5997:
8525:-dimensional" is to clarify the dimension of all manifolds in question, otherwise it is unclear whether a "5-dimensional cobordism" refers to a 5-dimensional cobordism between 4-dimensional manifolds or a 6-dimensional cobordism between 5-dimensional manifolds.
2216:
6293:
106:
that is closed, i.e., with empty boundary. In general, a closed manifold need not be a boundary: cobordism theory is the study of the difference between all closed manifolds and those that are boundaries. The theory was originally developed by
1944:
3656:). Conversely, given a handle decomposition of a cobordism, it comes from a suitable Morse function. In a suitably normalized setting this process gives a correspondence between handle decompositions and Morse functions on a cobordism.
8136:
8319:
424:
4898:
1517:
are used by some authors interchangeably; others distinguish them. When one wishes to distinguish the study of cobordism classes from the study of cobordisms as objects in their own right, one calls the equivalence question
4350:
6870:
3417:
1932:
5751:
2377:
2591:
3125:
There are two possible outcomes, depending on whether our gluing maps have the same or opposite orientation on the two boundary circles. If the orientations are the same (Fig. 2b), the resulting manifold is the
3572:
7522:
5162:
1228:
7799:
6056:
5830:
2208:
3511:
7364:
4588:
6966:
5237:
3631:
3123:
3076:
2884:
2717:
1807:
5891:
3171:
3029:
2975:
2931:
2837:
2670:
2152:
1368:
7025:
5884:
5364:
809:
774:
6177:
4783:
1493:
4163:
871:
2357:{\displaystyle \partial \left(\mathbb {S} ^{p}\times \mathbb {D} ^{q}\right)=\mathbb {S} ^{p}\times \mathbb {S} ^{q-1}=\partial \left(\mathbb {D} ^{p+1}\times \mathbb {S} ^{q-1}\right).}
6499:
4838:
7303:
4727:
4476:
6779:
5660:
7005:
4403:
1182:
8560:
a "cospan category": it is not the category of all cospans in "the category of manifolds with inclusions on the boundary", but rather a subcategory thereof, as the requirement that
3921:
1293:
4890:
527:
183:
4194:
4020:
3889:
8187:
7840:
7730:
7681:
7636:
7595:
6355:
6087:
3455:
6185:
1260:
315:
5266:
4223:
2775:
2746:
6613:
1650:
1614:
1399:
1324:
1143:
7992:
4657:
3754:
whose objects are closed manifolds and whose morphisms are cobordisms. Roughly speaking, composition is given by gluing together cobordisms end-to-end: the composition of (
8014:
6733:
6682:
2096:{\displaystyle N:=(M-\operatorname {int~im} \varphi )\cup _{\varphi |_{\mathbb {S} ^{p}\times \mathbb {S} ^{q-1}}}\left(\mathbb {D} ^{p+1}\times \mathbb {S} ^{q-1}\right)}
7335:
5340:
624:
490:
912:
188:
Cobordisms are studied both for the equivalence relation that they generate, and as objects in their own right. Cobordism is a much coarser equivalence relation than
8523:
4618:
656:
567:
6113:
8022:
4283:
4100:
3989:
5774:
736:
716:
696:
676:
467:
447:
4046:
6303:
Cobordism can also be defined for manifolds that have additional structure, notably an orientation. This is made formal in a general way using the notion of
8207:
5077:{\displaystyle \left\langle w_{i_{1}}(M)\cdots w_{i_{k}}(M),\right\rangle =\left\langle w_{i_{1}}(N)\cdots w_{i_{k}}(N),\right\rangle \in \mathbb {F} _{2}}
334:
6577:
9390:
4291:
8408:)) – while oriented cobordism is a product of Eilenberg–MacLane spectra rationally, and at 2, but not at odd primes: the oriented cobordism spectrum
6789:
1565:
The above is the most basic form of the definition. It is also referred to as unoriented bordism. In many situations, the manifolds in question are
3357:
1878:
2494:{\displaystyle W:=(M\times I)\cup _{\mathbb {S} ^{p}\times \mathbb {D} ^{q}\times \{1\}}\left(\mathbb {D} ^{p+1}\times \mathbb {D} ^{q}\right)}
5672:
1553:
are cobordant if they jointly bound a manifold; i.e., if their disjoint union is a boundary. Further, cobordism groups form an extraordinary
2531:
3711:. It performed an important role, historically speaking, in developments in topology in the 1950s and early 1960s, in particular in the
3524:
7478:
7011:(Wall, 1960). Two oriented manifolds are oriented cobordant if and only if their Stiefel–Whitney and Pontrjagin numbers are the same.
5090:
3838:
whose value on a disjoint union of manifolds is equivalent to the tensor product of its values on each of the constituent manifolds.
8325:
1187:
7733:
7743:
7465:{\displaystyle \sigma \left(\mathbb {P} ^{2i_{1}}(\mathbb {C} )\times \cdots \times \mathbb {P} ^{2i_{k}}(\mathbb {C} )\right)=1.}
6005:
8194:
5782:
3712:
2157:
5992:{\displaystyle \chi \left(\mathbb {P} ^{2i_{1}}(\mathbb {R} )\times \cdots \times \mathbb {P} ^{2i_{k}}(\mathbb {R} )\right)=1.}
3463:
4488:
6881:
5175:
3851:
3589:
3081:
3034:
2842:
2675:
1735:
1724:
Instead of considering additional structure, it is also possible to take into account various notions of manifold, especially
8918:
8787:
8752:
8595:
3132:
2990:
2936:
2892:
2798:
2631:
2113:
1329:
6624:
5835:
779:
744:
8324:
This is true for unoriented cobordism. Other cobordism theories do not reduce to ordinary homology in this way, notably
1009:. It is a 1-dimensional cobordism between the 0-dimensional manifolds {0}, {1}. More generally, for any closed manifold
208:
cannot be solved – but it is possible to classify manifolds up to cobordism. Cobordisms are central objects of study in
9024:
6120:
4732:
9378:
9373:
8935:
8628:
1455:
236:
8990:
8802:
8332:. The last-named theory in particular is much used by algebraic topologists as a computational tool (e.g., for the
5619:
This shows, for example, that every 3-dimensional closed manifold is the boundary of a 4-manifold (with boundary).
4109:
3823:
3735:
244:
817:
429:
Those points without a neighborhood homeomorphic to an open subset of
Euclidean space are the boundary points of
9368:
7553:
7545:
6736:
6002:
In particular such a product of real projective spaces is not null-cobordant. The mod 2 Euler characteristic map
3716:
3704:
8996:
6456:
4792:
3927:
with the disjoint union as operation. More specifically, if and denote the cobordism classes of the manifolds
9411:
8910:
8822:
8386:
7261:
4674:
4432:
2977:– that is, two disks - and it's clear that the result of doing so is to give us two disjoint spheres. (Fig. 2a)
196:
of manifolds, and is significantly easier to study and compute. It is not possible to classify manifolds up to
6749:
5628:
1433: + 1)-manifold. For example, the circle is null-cobordant since it bounds a disk. More generally, a
9270:
8878:
8719:
8145:
6978:
4365:
3894:
3203:
is a critical value with exactly one critical point in its preimage. If the index of this critical point is
7338:
4843:
503:
6288:{\displaystyle {\begin{cases}{\mathfrak {N}}\to \mathbb {F} _{2}\\\mapsto \chi (M)x^{\dim(M)}\end{cases}}}
153:
9416:
8873:
8714:
8454:
8197:
gives a starting point for calculations. The computation is only easy if the particular cobordism theory
8189:
can be effectively computed once one knows the cobordism theory of a point and the homology of the space
4168:
3994:
3863:
1077:
8151:
7804:
7694:
7645:
7600:
7559:
6629:
Oriented cobordism is the one of manifolds with an SO-structure. Equivalently, all manifolds need to be
6583:
The resulting cobordism groups are then defined analogously to the unoriented case. They are denoted by
6325:
6061:
3680:). Poincaré simultaneously defined both homology and cobordism, which are not the same, in general. See
3425:
1441: + 1)-disk. Also, every orientable surface is null-cobordant, because it is the boundary of a
1151:
9278:
8779:
8543:
8333:
1265:
1233:
288:
275:
5242:
4199:
2751:
2722:
8429:
6586:
3677:
1725:
1623:
1587:
116:
8031:
7967:
7337:
It is an oriented cobordism invariant, which is expressed in terms of the
Pontrjagin numbers by the
6194:
3750:
Cobordisms are objects of study in their own right, apart from cobordism classes. Cobordisms form a
2795:
For surgery on the 2-sphere, there are more possibilities, since we can start by cutting out either
9077:
7740:. The cobordism groups defined above are, from this point of view, the homology groups of a point:
6972:
4623:
3727:
1577:
and "cobordism with G-structure", respectively. Under favourable technical conditions these form a
7997:
6703:
6652:
9421:
9363:
9349:
7308:
7247:
5282:
3816:
2933:: If we remove a cylinder from the 2-sphere, we are left with two disks. We have to glue back in
1659:
When there is additional structure, the notion of cobordism must be formulated more precisely: a
579:
205:
8762:
Kosinski, Antoni A. (October 19, 2007). "Differential
Manifolds" (Document). Dover Publications.
1373:
1298:
1117:
1052:
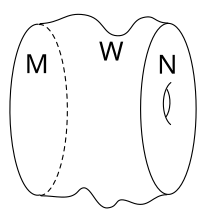
A cobordism between a single circle (at the top) and a pair of disjoint circles (at the bottom).
472:
9298:
9219:
9096:
9084:
9057:
9017:
8868:
7950:
3751:
3723:
3669:
318:
265:
9293:
1495:
is a (compact) closed manifold that is not the boundary of a manifold, as is explained below.
9140:
9067:
8947:
8775:
8709:
7008:
5347:
5169:
4414:
1450:
879:
326:
8741:
8131:{\displaystyle {\begin{cases}\Omega _{n}^{G}(X)\to H_{n}(X)\\(M,f)\mapsto f_{*}\end{cases}}}
9288:
9240:
9214:
9062:
8605:
8496:
8464:
6549:
5623:
4596:
3778:) is defined by gluing the right end of the first to the left end of the second, yielding (
3641:
3340:
1729:
629:
540:
120:
48:
6735:: both ends have opposite orientations. It is also the correct definition in the sense of
6092:
3636:
The Morse/Smale theorem states that for a Morse function on a cobordism, the flowlines of
8:
9135:
8474:
8382:
are very different, reflecting the difference between oriented and unoriented cobordism.
8361:
59:
9339:
4232:
4055:
3938:
9333:
9303:
9283:
9204:
9194:
9072:
9052:
8972:
8894:
8673:
8535:
8459:
6557:
5759:
2628:
As per the above definition, a surgery on the circle consists of cutting out a copy of
1703:
1506:
721:
701:
681:
661:
452:
432:
213:
209:
8314:{\displaystyle \Omega _{n}^{G}(X)=\sum _{p+q=n}H_{p}(X;\Omega _{q}^{G}({\text{pt}})).}
4025:
1048:
9328:
9321:
9187:
9145:
9010:
8964:
8914:
8811:
8783:
8748:
8665:
8624:
8591:
8365:
8329:
6366:
5269:
1714:
1692:
1617:
272:
9353:
8736:
6548:, the orthogonal group, giving back the unoriented cobordism, but also the subgroup
3665:
9101:
9047:
8956:
8843:
American
Mathematical Society Translations, Ser. 2, Vol. 11, pp. 1–114 (1959).
8657:
8449:
8425:
6370:
3827:
3739:
1699:
419:{\displaystyle \{(x_{1},\ldots ,x_{n})\in \mathbb {R} ^{n}\mid x_{n}\geqslant 0\}.}
8424:
In 1959, C.T.C. Wall proved that two manifolds are cobordant if and only if their
3258:
1502:
is to calculate the cobordism classes of manifolds subject to various conditions.
9160:
9155:
8856:
8601:
8587:
6320:
3696:
493:
283:
112:
9343:
4345:{\displaystyle {\mathfrak {N}}_{*}=\bigoplus _{n\geqslant 0}{\mathfrak {N}}_{n}}
325:
is allowed to have a neighborhood that is homeomorphic to an open subset of the
9250:
9182:
8846:
8836:
8725:
8469:
6742:
Unlike in the unoriented cobordism group, where every element is two-torsion, 2
4422:
4356:
3688:
3192:
2107:
1710:
232:
197:
189:
77:
6865:{\displaystyle \Omega _{*}^{\text{SO}}\otimes \mathbb {Q} =\mathbb {Q} \left,}
1616:, with grading by dimension, addition by disjoint union and multiplication by
926:
if such a cobordism exists. All manifolds cobordant to a fixed given manifold
9405:
9260:
9170:
9150:
8968:
8942:
8815:
8705:
8669:
8340:
7541:
6630:
6565:
6312:
3924:
2601:
1566:
1146:
1000:
497:
279:
201:
193:
52:
6505:-structure gives rise to a more general notion of cobordism. In particular,
6179:, these group homomorphism assemble into a homomorphism of graded algebras:
1717:: such a process changes a normal map to another normal map within the same
9245:
9165:
9111:
6649:
for clarity) are such that the boundary (with the induced orientations) is
6362:
3831:
3700:
3174:
221:
64:
8927:
6746:
is not in general an oriented boundary, that is, 2 ≠ 0 when considered in
4479:
3692:
3412:{\displaystyle W=\mathbb {S} ^{1}\times \mathbb {D} ^{2}-\mathbb {D} ^{3}}
2605:
1927:{\displaystyle \varphi :\mathbb {S} ^{p}\times \mathbb {D} ^{q}\subset M,}
1825:
are manifolds with boundary, then the boundary of the product manifold is
108:
9255:
8884:
8797:
8694:
8441:
8374:. Note that even for similar groups, Thom spectra can be very different:
6537:
6308:
2719:
The pictures in Fig. 1 show that the result of doing this is either (i)
2609:
1578:
1570:
1102:
The pair of pants is an example of a more general cobordism: for any two
1083:(see the figure at right). Thus the pair of pants is a cobordism between
225:
40:
8827:
Methods of algebraic topology from the point of view of cobordism theory
8385:
From the point of view of spectra, unoriented cobordism is a product of
5746:{\displaystyle \chi _{\partial W}=\left(1-(-1)^{\dim W}\right)\chi _{W}}
9199:
9130:
9089:
8976:
8677:
8645:
8351:
7684:
6561:
4022:
into an abelian group. The identity element of this group is the class
1442:
5666:
is an unoriented cobordism invariant. This is implied by the equation
8767:
6692:
with the reversed orientation. For example, boundary of the cylinder
2586:{\displaystyle \mathbb {D} ^{p+1}\times \mathbb {S} ^{q-1}\subset N.}
1873:
739:
574:
8960:
8661:
8201:, in which case the bordism groups are the ordinary homology groups
8198:
8148:
apart from the dimension axiom. This does not mean that the groups
3681:
3303:
is Morse and such that all critical points occur in the interior of
2600:
Every cobordism is a union of elementary cobordisms, by the work of
1653:
1536:
9209:
9177:
9126:
9033:
8851:
On the formal group laws of unoriented and complex cobordism theory
7549:
6875:
the polynomial algebra generated by the oriented cobordism classes
6298:
3731:
3708:
1184:
The previous example is a particular case, since the connected sum
231:
are fundamental in the study of high-dimensional manifolds, namely
92:
55:
8913:(ed.). World Scientific Publishing Co. Pte. Ltd., Hackensack, NJ.
7535:
2981:
2787:
2779:
8556:
While every cobordism is a cospan, the category of cobordisms is
3835:
3567:{\displaystyle \mathbb {S} ^{0}\times \mathbb {D} ^{2}\subset M,}
2620:
1718:
7517:{\displaystyle \sigma :\Omega _{4i}^{\text{SO}}\to \mathbb {Z} }
5157:{\displaystyle w_{i}(M)\in H^{i}\left(M;\mathbb {F} _{2}\right)}
1809:, which are harder to compute than the differentiable variants.
953:
to be compact in the definition of cobordism. Note however that
3800:
3703:
construction. Cobordism theory became part of the apparatus of
3420:
3259:
Geometry, and the connection with Morse theory and handlebodies
1061:
8907:
Topological library. Part 1: cobordisms and their applications
4229:. The cartesian product of manifolds defines a multiplication
1541:, meaning boundary. Hence bordism is the study of boundaries.
1223:{\displaystyle \mathbb {S} ^{1}\mathbin {\#} \mathbb {S} ^{1}}
8772:
The classifying spaces for surgery and cobordism of manifolds
4226:
3458:
3127:
7794:{\displaystyle \Omega _{n}^{G}=\Omega _{n}^{G}({\text{pt}})}
6051:{\displaystyle \chi :{\mathfrak {N}}_{2i}\to \mathbb {Z} /2}
3350:
658:-dimensional compact differentiable manifold with boundary,
235:. In algebraic topology, cobordism theories are fundamental
115:(i.e., differentiable), but there are now also versions for
19:
9002:
8743:
A history of algebraic and differential topology, 1900–1960
8124:
6281:
5832:
is a well-defined group homomorphism. For example, for any
5825:{\displaystyle \chi :{\mathfrak {N}}_{i}\to \mathbb {Z} /2}
3695:
showed that cobordism groups could be computed by means of
3691:
in geometric work on manifolds. It came to prominence when
2203:{\displaystyle \mathbb {D} ^{p+1}\times \mathbb {S} ^{q-1}}
8841:
Smooth manifolds and their applications in homotopy theory
8144:
The bordism and cobordism theories of a space satisfy the
6783:
The oriented cobordism groups are given modulo torsion by
3506:{\displaystyle N=\mathbb {S} ^{1}\times \mathbb {S} ^{1},}
3311:
is called a Morse function on a cobordism. The cobordism (
1569:, or carry some other additional structure referred to as
8955:(2). The Annals of Mathematics, Vol. 72, No. 2: 292–311.
8932:
Quelques propriétés globales des variétés différentiables
8016:
in the oriented case), defining a natural transformation
7014:
The low-dimensional oriented cobordism groups are :
4583:{\displaystyle {\mathfrak {N}}_{*}=\mathbb {F} _{2}\left}
3323:) is a union of the traces of a sequence of surgeries on
3255:) that can be identified with the trace of this surgery.
72:) of a manifold. Two manifolds of the same dimension are
7258:
is defined as the signature of the intersection form on
6961:{\displaystyle y_{4i}=\left\in \Omega _{4i}^{\text{SO}}}
5232:{\displaystyle \in H_{n}\left(M;\mathbb {F} _{2}\right)}
3626:{\displaystyle \mathbb {D} ^{1}\times \mathbb {D} ^{2}.}
3118:{\displaystyle \mathbb {S} ^{1}\times \mathbb {D} ^{1}.}
3071:{\displaystyle \mathbb {S} ^{0}\times \mathbb {D} ^{2},}
2879:{\displaystyle \mathbb {S} ^{1}\times \mathbb {D} ^{1}.}
2712:{\displaystyle \mathbb {D} ^{1}\times \mathbb {S} ^{0}.}
1802:{\displaystyle \Omega _{*}^{PL}(X),\Omega _{*}^{TOP}(X)}
8861:
Complex cobordism and stable homotopy groups of spheres
8621:
Complex cobordism and stable homotopy groups of spheres
6536:) is some group homomorphism. This is referred to as a
3852:
List of cohomology theories § Unoriented cobordism
3199: + 1)-dimensional manifold, and suppose that
3166:{\displaystyle \mathbb {S} ^{1}\times \mathbb {S} ^{1}}
3024:{\displaystyle \mathbb {S} ^{0}\times \mathbb {D} ^{2}}
2970:{\displaystyle \mathbb {S} ^{0}\times \mathbb {D} ^{2}}
2926:{\displaystyle \mathbb {S} ^{1}\times \mathbb {D} ^{1}}
2832:{\displaystyle \mathbb {S} ^{0}\times \mathbb {D} ^{2}}
2665:{\displaystyle \mathbb {S} ^{0}\times \mathbb {D} ^{1}}
2147:{\displaystyle \mathbb {S} ^{p}\times \mathbb {D} ^{q}}
1363:{\displaystyle \mathbb {S} ^{0}\times \mathbb {D} ^{n}}
58:
of the same dimension, set up using the concept of the
4421:, which depend on the stable isomorphism class of the
8853:
Bull. Amer. Math. Soc., 75 (1969) pp. 1293–1298.
8499:
8210:
8154:
8025:
8000:
7970:
7807:
7746:
7697:
7648:
7603:
7562:
7481:
7367:
7311:
7264:
7023:
6981:
6884:
6792:
6752:
6706:
6655:
6625:
List of cohomology theories § Oriented cobordism
6589:
6459:
6328:
6188:
6123:
6095:
6064:
6008:
5894:
5838:
5785:
5762:
5675:
5631:
5362:
5285:
5245:
5178:
5093:
4901:
4846:
4795:
4735:
4677:
4626:
4599:
4491:
4435:
4368:
4294:
4235:
4202:
4171:
4112:
4058:
4028:
3997:
3941:
3897:
3866:
3592:
3527:
3466:
3428:
3360:
3135:
3084:
3037:
2993:
2939:
2895:
2845:
2801:
2754:
2725:
2678:
2634:
2534:
2380:
2219:
2160:
2116:
1947:
1881:
1738:
1626:
1590:
1505:
Null-cobordisms with additional structure are called
1458:
1376:
1332:
1301:
1268:
1236:
1190:
1154:
1120:
949: × [0, 1); for this reason we require
882:
820:
782:
747:
724:
704:
684:
664:
632:
582:
543:
506:
475:
455:
435:
337:
291:
156:
8704:
7007:
is determined by the
Stiefel–Whitney and Pontrjagin
5353:
The low-dimensional unoriented cobordism groups are
8732:
Proc. Camb. Phil. Soc. 57, pp. 200–208 (1961).
8141:which is far from being an isomorphism in general.
5879:{\displaystyle i_{1},\cdots ,i_{k}\in \mathbb {N} }
3734:played a basic role in the Atiyah–Segal axioms for
3684:for the relationship between bordism and homology.
3664:Cobordism had its roots in the (failed) attempt by
804:{\displaystyle j\colon N\hookrightarrow \partial W}
769:{\displaystyle i\colon M\hookrightarrow \partial W}
8740:
8517:
8313:
8199:reduces to a product of ordinary homology theories
8181:
8130:
8008:
7986:
7834:
7793:
7724:
7675:
7630:
7589:
7516:
7464:
7329:
7297:
7235:
6999:
6960:
6864:
6773:
6727:
6676:
6607:
6493:
6349:
6287:
6171:
6107:
6081:
6050:
5991:
5878:
5824:
5768:
5745:
5654:
5608:
5334:
5260:
5231:
5156:
5076:
4884:
4832:
4777:
4721:
4651:
4612:
4582:
4470:
4397:
4344:
4277:
4217:
4188:
4157:
4094:
4052:-manifolds which are boundaries. Further we have
4040:
4014:
3983:
3915:
3883:
3856:The set of cobordism classes of closed unoriented
3625:
3566:
3505:
3449:
3411:
3165:
3117:
3070:
3023:
2985:Fig. 2c. This shape cannot be embedded in 3-space.
2969:
2925:
2878:
2831:
2769:
2740:
2711:
2664:
2585:
2493:
2356:
2202:
2146:
2095:
1926:
1801:
1644:
1608:
1487:
1393:
1362:
1318:
1287:
1254:
1222:
1176:
1137:
906:
865:
803:
768:
730:
710:
690:
670:
650:
618:
561:
521:
484:
461:
441:
418:
309:
177:
6501:. Considering only manifolds and cobordisms with
6172:{\displaystyle \chi (M\times N)=\chi (M)\chi (N)}
1401:, and the cobordism is the trace of the surgery.
217:
9403:
8909:. Series on Knots and Everything. Vol. 39.
6299:Cobordism of manifolds with additional structure
4778:{\displaystyle \left(i_{1},\cdots ,i_{k}\right)}
8945:(1960). "Determination of the cobordism ring".
8889:On Thom spectra, orientability, and (co)bordism
7536:Cobordism as an extraordinary cohomology theory
3991:; this is a well-defined operation which turns
3830:from a category of cobordisms to a category of
3682:Cobordism as an extraordinary cohomology theory
1488:{\displaystyle \mathbb {P} ^{2n}(\mathbb {R} )}
1099:is given by the disjoint union of three disks.
961:required to be connected; as a consequence, if
8866:
9018:
8766:
8586:, Classics in Mathematics, Berlin, New York:
7552:. Similarly, every cobordism theory Ω has an
4158:{\displaystyle M\sqcup M=\partial (M\times )}
3860:-dimensional manifolds is usually denoted by
1437:-sphere is null-cobordant since it bounds a (
2443:
2437:
1534:
945:is the boundary of the non-compact manifold
866:{\displaystyle \partial W=i(M)\sqcup j(N)~.}
410:
338:
84:of a compact manifold one dimension higher.
6975:(Thom, 1952). The oriented cobordism group
4359:, with the grading given by the dimension.
1425:and the empty manifold; in other words, if
999:The simplest example of a cobordism is the
9386:
9359:
9025:
9011:
6494:{\displaystyle {\tilde {\nu }}:M\to X_{k}}
4833:{\displaystyle i\geqslant 1,i\neq 2^{j}-1}
4593:the polynomial algebra with one generator
876:The terminology is usually abbreviated to
8735:
8350:, the Thom spectrum is composed from the
8002:
7972:
7510:
7444:
7420:
7402:
7378:
7298:{\displaystyle H^{2i}(M)\in \mathbb {Z} }
7291:
7216:
7181:
7051:
6925:
6908:
6820:
6812:
6401:). Given a collection of spaces and maps
6331:
6209:
6072:
6036:
5971:
5947:
5929:
5905:
5872:
5810:
5648:
5590:
5549:
5533:
5461:
5389:
5320:
5306:
5248:
5214:
5139:
5064:
4722:{\displaystyle =\in {\mathfrak {N}}_{n},}
4511:
4471:{\displaystyle =0\in {\mathfrak {N}}_{n}}
4429:has a stably trivial tangent bundle then
4205:
3673:
3610:
3595:
3545:
3530:
3490:
3475:
3437:
3399:
3384:
3369:
3173:but if they are different, we obtain the
3153:
3138:
3102:
3087:
3055:
3040:
3011:
2996:
2957:
2942:
2913:
2898:
2863:
2848:
2819:
2804:
2757:
2728:
2696:
2681:
2652:
2637:
2558:
2537:
2476:
2455:
2424:
2409:
2330:
2309:
2280:
2265:
2245:
2230:
2184:
2163:
2134:
2119:
2072:
2051:
2024:
2009:
1905:
1890:
1522:, and the study of cobordisms as objects
1478:
1461:
1350:
1335:
1239:
1210:
1193:
381:
294:
8904:
8800:(1962). "A survey of cobordism theory".
8761:
8699:Stable homotopy and generalised homology
8584:Algebraic topology—homotopy and homology
7691:, and the generalized cohomology groups
6774:{\displaystyle \Omega _{*}^{\text{SO}}.}
6576:, and the trivial group, giving rise to
5655:{\displaystyle \chi (M)\in \mathbb {Z} }
3349:
2980:
2786:
2778:
2619:
1047:
240:
216:. In geometric topology, cobordisms are
142:whose boundary is the disjoint union of
18:
8618:
8581:
7000:{\displaystyle \Omega _{*}^{\text{SO}}}
5756:for any compact manifold with boundary
4398:{\displaystyle \in {\mathfrak {N}}_{n}}
3845:
3299:. By general position, one can assume
1812:
1574:
9404:
8796:
8339:Cobordism theories are represented by
4892:the Stiefel-Whitney numbers are equal
3916:{\displaystyle \Omega _{n}^{\text{O}}}
3745:
1709:In a similar vein, a standard tool in
9006:
8646:"Determination of the Cobordism Ring"
8534:
6618:
6365:, which in turn is a subspace of the
6319:into a sufficiently high-dimensional
4885:{\displaystyle i_{1}+\cdots +i_{k}=n}
4413:is determined by the Stiefel–Whitney
3687:Bordism was explicitly introduced by
522:{\displaystyle \partial M=\emptyset }
8941:
8643:
8568:form a partition of the boundary of
7597:and cohomology ("cobordism") groups
1732:. This gives rise to bordism groups
1295:is obtained from the disjoint union
178:{\displaystyle \partial W=M\sqcup N}
8195:Atiyah–Hirzebruch spectral sequence
7556:, with homology ("bordism") groups
7544:theory (real, complex etc.) has an
6453:-structure is a lift of ν to a map
6199:
6018:
5795:
5662:modulo 2 of an unoriented manifold
5571:
5514:
5483:
5442:
5411:
5370:
4729:if and only if for each collection
4705:
4495:
4457:
4384:
4331:
4298:
4189:{\displaystyle {\mathfrak {N}}_{n}}
4175:
4015:{\displaystyle {\mathfrak {N}}_{n}}
4001:
3884:{\displaystyle {\mathfrak {N}}_{n}}
3870:
3207: + 1, then the level-set
1076:together make up the boundary of a
321:is similar, except that a point of
13:
8280:
8212:
8182:{\displaystyle \Omega _{G}^{n}(X)}
8156:
8035:
7835:{\displaystyle \Omega _{n}^{G}(X)}
7809:
7766:
7748:
7725:{\displaystyle \Omega _{G}^{*}(X)}
7699:
7676:{\displaystyle \Omega _{*}^{G}(X)}
7650:
7642:. The generalized homology groups
7631:{\displaystyle \Omega _{G}^{n}(X)}
7605:
7590:{\displaystyle \Omega _{n}^{G}(X)}
7564:
7489:
7193:
7159:
7127:
7095:
7063:
7029:
6983:
6941:
6794:
6754:
6591:
6433:) (compatible with the inclusions
6350:{\displaystyle \mathbb {R} ^{n+k}}
6082:{\displaystyle i\in \mathbb {N} ,}
5681:
4125:
4086:
4032:
3899:
3450:{\displaystyle M=\mathbb {S} ^{2}}
3182:
2299:
2220:
2110:, via cutting out the interior of
1979:
1976:
1970:
1967:
1964:
1770:
1740:
1628:
1592:
1273:
1204:
1177:{\displaystyle M\mathbin {\#} M'.}
1159:
821:
795:
760:
516:
507:
476:
245:topological quantum field theories
157:
14:
9433:
8984:
8936:Commentarii Mathematici Helvetici
8829:, Izv. Akad. Nauk SSSR Ser. Mat.
8708:; Voitsekhovskii, M. I. (2001) ,
3891:(rather than the more systematic
3715:, and in the first proofs of the
3327:, one for each critical point of
3275:) there exists a smooth function
3219: + ε) is obtained from
1687:= SO for oriented cobordism, and
1288:{\displaystyle M\mathbin {\#} M'}
1255:{\displaystyle \mathbb {S} ^{1}.}
310:{\displaystyle \mathbb {R} ^{n}.}
237:extraordinary cohomology theories
9385:
9358:
9348:
9338:
9327:
9317:
9316:
9110:
8419:
8412:is rather more complicated than
5261:{\displaystyle \mathbb {F} _{2}}
4218:{\displaystyle \mathbb {F} _{2}}
3824:topological quantum field theory
3738:, which is an important part of
3736:topological quantum field theory
3730:and cobordisms between these as
2770:{\displaystyle \mathbb {S} ^{1}}
2741:{\displaystyle \mathbb {S} ^{1}}
1652:are the coefficient groups of a
1429:is the entire boundary of some (
1421:if there is a cobordism between
204:in dimensions ≥ 4 – because the
8901:, Princeton Univ. Press (1968).
7898:if there exists a G-cobordism (
7554:extraordinary cohomology theory
7546:extraordinary cohomology theory
6737:extraordinary cohomology theory
6608:{\displaystyle \Omega _{*}^{G}}
3713:Hirzebruch–Riemann–Roch theorem
3705:extraordinary cohomology theory
1645:{\displaystyle \Omega _{*}^{G}}
1609:{\displaystyle \Omega _{*}^{G}}
811:with disjoint images such that
8905:Taimanov, Iskander A. (2007).
8637:
8612:
8575:
8550:
8528:
8512:
8500:
8487:
8305:
8302:
8294:
8270:
8232:
8226:
8176:
8170:
8118:
8112:
8099:
8096:
8084:
8077:
8071:
8058:
8055:
8049:
7987:{\displaystyle \mathbb {Z} /2}
7829:
7823:
7788:
7780:
7719:
7713:
7670:
7664:
7625:
7619:
7584:
7578:
7506:
7448:
7440:
7406:
7398:
7321:
7315:
7284:
7278:
6929:
6921:
6722:
6713:
6671:
6662:
6478:
6466:
6273:
6267:
6253:
6247:
6241:
6238:
6232:
6225:
6219:
6204:
6166:
6160:
6154:
6148:
6139:
6127:
6032:
5975:
5967:
5933:
5925:
5806:
5713:
5703:
5641:
5635:
5324:
5316:
5185:
5179:
5110:
5104:
5051:
5045:
5039:
5033:
5010:
5004:
4971:
4965:
4959:
4953:
4930:
4924:
4696:
4690:
4684:
4678:
4537:
4442:
4436:
4375:
4369:
4269:
4257:
4251:
4245:
4242:
4236:
4152:
4149:
4137:
4128:
4089:
4083:
4077:
4071:
4065:
4059:
4035:
4029:
3978:
3966:
3960:
3954:
3948:
3942:
3672:purely in terms of manifolds (
2399:
2387:
2002:
1989:
1954:
1796:
1790:
1763:
1757:
1683:= O for unoriented cobordism,
1482:
1474:
1404:
1326:by surgery on an embedding of
1091:. A simpler cobordism between
901:
883:
854:
848:
839:
833:
792:
757:
645:
633:
613:
583:
556:
544:
373:
341:
1:
8701:, Univ. Chicago Press (1974).
8688:
5342:, the cobordism class of the
4659:. Thus two unoriented closed
4652:{\displaystyle i\neq 2^{j}-1}
3078:we glue back in the cylinder
2748:again, or (ii) two copies of
2615:
532:
250:
9032:
8770:; Milgram, R. James (1979).
8619:Ravenel, D.C. (April 1986).
8009:{\displaystyle \mathbb {Z} }
7528:≥ 1, and an isomorphism for
7339:Hirzebruch signature theorem
6728:{\displaystyle M\sqcup (-M)}
6677:{\displaystyle M\sqcup (-N)}
6089:and a group isomorphism for
3799:). A cobordism is a kind of
3354:The 3-dimensional cobordism
3235:-surgery. The inverse image
1702:. Many more are detailed by
255:
91: + 1)-dimensional
7:
8874:Encyclopedia of Mathematics
8803:L'Enseignement Mathématique
8715:Encyclopedia of Mathematics
8582:Switzer, Robert M. (2002),
8455:List of cohomology theories
8435:
7330:{\displaystyle \sigma (M).}
5335:{\displaystyle x_{i}=\left}
3717:Atiyah–Singer index theorem
3343:for each critical point of
3031:: Having cut out two disks
1817:Recall that in general, if
1654:generalised homology theory
1560:
994:
619:{\displaystyle (W;M,N,i,j)}
500:manifold without boundary (
10:
9438:
9279:Banach fixed-point theorem
8780:Princeton University Press
8544:Princeton University Press
8334:homotopy groups of spheres
8328:, oriented cobordism and
7951:fundamental homology class
6622:
3849:
3726:with compact manifolds as
3659:
1545:means "jointly bound", so
1445:. On the other hand, the 2
1394:{\displaystyle M\sqcup M'}
1319:{\displaystyle M\sqcup M'}
1138:{\displaystyle M\sqcup M'}
485:{\displaystyle \partial M}
9312:
9269:
9233:
9119:
9108:
9040:
8899:Notes on cobordism theory
8737:Dieudonné, Jean Alexandre
8540:Notes on cobordism theory
8387:Eilenberg–MacLane spectra
8146:Eilenberg–Steenrod axioms
6973:complex projective spaces
6357:gives rise to a map from
5279:it is possible to choose
4048:consisting of all closed
1679:. The basic examples are
8867:Yuli B. Rudyak (2001) ,
8480:
7964:) (with coefficients in
3935:respectively, we define
3586:by attaching a 1-handle
3243:() defines a cobordism (
278:(i.e., near each point)
241:categories of cobordisms
8644:Wall, C. T. C. (1960).
8572:is a global constraint.
8362:standard vector bundles
7866:(with G-structure) and
6645:) (also referred to as
4663:-dimensional manifolds
4405:of a closed unoriented
4227:field with two elements
4196:is a vector space over
3817:dagger compact category
1620:. The cobordism groups
1524:cobordisms of manifolds
1106:-dimensional manifolds
907:{\displaystyle (W;M,N)}
206:word problem for groups
102:-dimensional manifold ∂
9334:Mathematics portal
9234:Metrics and properties
9220:Second-countable space
8999:on the Manifold Atlas.
8993:on the Manifold Atlas.
8747:. Boston: Birkhäuser.
8519:
8315:
8183:
8132:
8010:
7988:
7945:-dimensional manifold
7862:-dimensional manifold
7836:
7795:
7726:
7677:
7632:
7591:
7518:
7466:
7331:
7299:
7254:-dimensional manifold
7237:
7009:characteristic numbers
7001:
6962:
6866:
6775:
6729:
6678:
6609:
6495:
6351:
6289:
6173:
6109:
6083:
6052:
5993:
5880:
5826:
5770:
5747:
5656:
5610:
5336:
5262:
5233:
5158:
5078:
4886:
4834:
4779:
4723:
4653:
4614:
4584:
4472:
4415:characteristic numbers
4409:-dimensional manifold
4399:
4346:
4279:
4219:
4190:
4159:
4096:
4042:
4016:
3985:
3917:
3885:
3633:
3627:
3568:
3507:
3451:
3413:
3167:
3119:
3072:
3025:
2986:
2971:
2927:
2880:
2833:
2792:
2784:
2771:
2742:
2713:
2666:
2625:
2587:
2495:
2358:
2204:
2148:
2097:
1928:
1856:Now, given a manifold
1803:
1646:
1610:
1535:
1489:
1395:
1364:
1320:
1289:
1256:
1224:
1178:
1139:
1114:′, the disjoint union
1053:
1037:) is a cobordism from
941:Every closed manifold
908:
867:
805:
770:
732:
712:
692:
672:
652:
620:
563:
523:
486:
463:
443:
420:
319:manifold with boundary
311:
179:
138:is a compact manifold
36:
16:Concept in mathematics
9412:Differential topology
8948:Annals of Mathematics
8863:, Acad. Press (1986).
8776:Princeton, New Jersey
8730:Bordism and cobordism
8650:Annals of Mathematics
8520:
8518:{\displaystyle (n+1)}
8316:
8184:
8133:
8011:
7989:
7922:, which restricts to
7837:
7796:
7727:
7678:
7633:
7592:
7519:
7467:
7344:For example, for any
7332:
7300:
7238:
7002:
6963:
6867:
6776:
6730:
6679:
6623:Further information:
6610:
6496:
6352:
6315:ν of an immersion of
6311:). Very briefly, the
6290:
6174:
6117:Moreover, because of
6110:
6084:
6053:
5994:
5881:
5827:
5771:
5748:
5657:
5611:
5348:real projective space
5337:
5263:
5234:
5170:Stiefel-Whitney class
5159:
5079:
4887:
4835:
4780:
4724:
4654:
4615:
4613:{\displaystyle x_{i}}
4585:
4473:
4400:
4347:
4280:
4220:
4191:
4160:
4097:
4043:
4017:
3986:
3918:
3886:
3850:Further information:
3815:. The category is a
3628:
3569:
3508:
3452:
3414:
3353:
3231: − ε) by a
3168:
3120:
3073:
3026:
2984:
2972:
2928:
2881:
2834:
2790:
2782:
2772:
2743:
2714:
2667:
2623:
2595:reversing the surgery
2588:
2496:
2359:
2210:along their boundary
2205:
2149:
2098:
1929:
1804:
1730:topological manifolds
1726:piecewise linear (PL)
1647:
1611:
1573:. This gives rise to
1490:
1451:real projective space
1396:
1365:
1321:
1290:
1257:
1225:
1179:
1140:
1051:
909:
868:
806:
771:
733:
713:
693:
673:
653:
651:{\displaystyle (n+1)}
621:
564:
562:{\displaystyle (n+1)}
524:
496:is, by definition, a
487:
464:
444:
421:
312:
282:to an open subset of
260:Roughly speaking, an
180:
121:topological manifolds
22:
9289:Invariance of domain
9241:Euler characteristic
9215:Bundle (mathematics)
8497:
8465:Cobordism hypothesis
8208:
8152:
8023:
7998:
7968:
7805:
7744:
7695:
7646:
7601:
7560:
7479:
7365:
7309:
7262:
7021:
6979:
6882:
6790:
6750:
6704:
6653:
6587:
6457:
6326:
6186:
6121:
6108:{\displaystyle i=1.}
6093:
6062:
6006:
5892:
5836:
5783:
5760:
5673:
5629:
5624:Euler characteristic
5360:
5283:
5243:
5176:
5091:
4899:
4844:
4793:
4789:-tuples of integers
4733:
4675:
4624:
4597:
4489:
4433:
4366:
4362:The cobordism class
4292:
4233:
4200:
4169:
4110:
4056:
4026:
3995:
3939:
3895:
3864:
3846:Unoriented cobordism
3590:
3525:
3464:
3426:
3358:
3339:× by attaching one
3133:
3082:
3035:
2991:
2937:
2893:
2843:
2799:
2752:
2723:
2676:
2632:
2532:
2378:
2217:
2158:
2114:
1945:
1879:
1813:Surgery construction
1736:
1624:
1588:
1575:"oriented cobordism"
1520:bordism of manifolds
1456:
1374:
1330:
1299:
1266:
1234:
1188:
1152:
1145:is cobordant to the
1118:
880:
818:
780:
745:
722:
702:
682:
662:
630:
580:
541:
504:
473:
453:
433:
335:
289:
218:intimately connected
154:
87:The boundary of an (
49:equivalence relation
9299:Tychonoff's theorem
9294:Poincaré conjecture
9048:General (point-set)
8475:Timeline of bordism
8293:
8225:
8169:
8048:
7994:in general, and in
7878:a map. Such pairs (
7822:
7779:
7761:
7712:
7663:
7618:
7577:
7505:
7206:
7172:
7140:
7108:
7076:
7042:
6996:
6957:
6807:
6767:
6647:oriented cobordisms
6604:
6540:. Examples include
3912:
3834:. That is, it is a
3746:Categorical aspects
3642:handle presentation
3263:Given a cobordism (
1789:
1756:
1641:
1605:
243:are the domains of
9417:Algebraic topology
9284:De Rham cohomology
9205:Polyhedral complex
9195:Simplicial complex
8891:, Springer (2008).
8623:. Academic Press.
8515:
8460:Symplectic filling
8426:Pontrjagin numbers
8366:classifying spaces
8311:
8279:
8259:
8211:
8179:
8155:
8128:
8123:
8034:
8006:
7984:
7846:classes of pairs (
7832:
7808:
7791:
7765:
7747:
7722:
7698:
7673:
7649:
7628:
7604:
7587:
7563:
7514:
7488:
7475:The signature map
7462:
7327:
7305:and is denoted by
7295:
7233:
7231:
7192:
7158:
7126:
7094:
7062:
7028:
6997:
6982:
6958:
6940:
6862:
6793:
6771:
6753:
6725:
6674:
6619:Oriented cobordism
6605:
6590:
6558:oriented cobordism
6491:
6347:
6285:
6280:
6169:
6105:
6079:
6048:
5989:
5876:
5822:
5766:
5743:
5652:
5606:
5604:
5332:
5258:
5229:
5154:
5074:
4882:
4830:
4775:
4719:
4649:
4620:in each dimension
4610:
4580:
4468:
4395:
4342:
4327:
4278:{\displaystyle =,}
4275:
4215:
4186:
4155:
4095:{\displaystyle +=}
4092:
4038:
4012:
3984:{\displaystyle +=}
3981:
3913:
3898:
3881:
3668:in 1895 to define
3634:
3623:
3564:
3503:
3447:
3409:
3307:. In this setting
3163:
3115:
3068:
3021:
2987:
2967:
2923:
2876:
2829:
2793:
2785:
2767:
2738:
2709:
2662:
2626:
2583:
2491:
2354:
2200:
2144:
2093:
1924:
1799:
1769:
1739:
1642:
1627:
1606:
1591:
1533:comes from French
1485:
1391:
1360:
1316:
1285:
1262:The connected sum
1252:
1220:
1174:
1135:
1054:
904:
863:
801:
766:
728:
708:
688:
668:
648:
616:
559:
519:
482:
459:
449:; the boundary of
439:
416:
307:
214:algebraic topology
210:geometric topology
175:
130:between manifolds
47:is a fundamental
37:
9399:
9398:
9188:fundamental group
8951:. Second Series.
8938:28, 17-86 (1954).
8920:978-981-270-559-4
8789:978-0-691-08226-4
8754:978-0-8176-3388-2
8726:Michael F. Atiyah
8706:Anosov, Dmitri V.
8597:978-3-540-42750-6
8542:. Princeton, NJ:
8330:complex cobordism
8300:
8238:
7786:
7503:
7204:
7170:
7138:
7106:
7074:
7040:
6994:
6955:
6805:
6765:
6556:, giving rise to
6469:
6367:classifying space
5769:{\displaystyle W}
5270:fundamental class
4312:
3910:
3722:In the 1980s the
3640:′ give rise to a
3335:is obtained from
2524:is obtained from
1975:
1700:complex manifolds
1693:complex cobordism
1618:cartesian product
1557:, hence the co-.
1555:cohomology theory
1230:is isomorphic to
859:
731:{\displaystyle N}
711:{\displaystyle M}
691:{\displaystyle n}
671:{\displaystyle W}
626:consisting of an
462:{\displaystyle M}
442:{\displaystyle M}
273:topological space
9429:
9389:
9388:
9362:
9361:
9352:
9342:
9332:
9331:
9320:
9319:
9114:
9027:
9020:
9013:
9004:
9003:
8980:
8924:
8881:
8833:(1967), 855–951.
8819:
8793:
8763:
8758:
8746:
8722:
8695:John Frank Adams
8682:
8681:
8641:
8635:
8634:
8616:
8610:
8608:
8579:
8573:
8554:
8548:
8547:
8536:Stong, Robert E.
8532:
8526:
8524:
8522:
8521:
8516:
8491:
8450:Link concordance
8400:
8346:: given a group
8326:framed cobordism
8320:
8318:
8317:
8312:
8301:
8298:
8292:
8287:
8269:
8268:
8258:
8224:
8219:
8188:
8186:
8185:
8180:
8168:
8163:
8137:
8135:
8134:
8129:
8127:
8126:
8111:
8110:
8070:
8069:
8047:
8042:
8015:
8013:
8012:
8007:
8005:
7993:
7991:
7990:
7985:
7980:
7975:
7842:is the group of
7841:
7839:
7838:
7833:
7821:
7816:
7800:
7798:
7797:
7792:
7787:
7784:
7778:
7773:
7760:
7755:
7731:
7729:
7728:
7723:
7711:
7706:
7682:
7680:
7679:
7674:
7662:
7657:
7637:
7635:
7634:
7629:
7617:
7612:
7596:
7594:
7593:
7588:
7576:
7571:
7524:is onto for all
7523:
7521:
7520:
7515:
7513:
7504:
7501:
7499:
7471:
7469:
7468:
7463:
7455:
7451:
7447:
7439:
7438:
7437:
7436:
7423:
7405:
7397:
7396:
7395:
7394:
7381:
7336:
7334:
7333:
7328:
7304:
7302:
7301:
7296:
7294:
7277:
7276:
7250:of an oriented 4
7242:
7240:
7239:
7234:
7232:
7225:
7224:
7219:
7205:
7202:
7200:
7184:
7171:
7168:
7166:
7139:
7136:
7134:
7107:
7104:
7102:
7075:
7072:
7070:
7054:
7041:
7038:
7036:
7006:
7004:
7003:
6998:
6995:
6992:
6990:
6967:
6965:
6964:
6959:
6956:
6953:
6951:
6936:
6932:
6928:
6920:
6919:
6911:
6897:
6896:
6871:
6869:
6868:
6863:
6858:
6854:
6841:
6840:
6823:
6815:
6806:
6803:
6801:
6780:
6778:
6777:
6772:
6766:
6763:
6761:
6734:
6732:
6731:
6726:
6683:
6681:
6680:
6675:
6633:and cobordisms (
6614:
6612:
6611:
6606:
6603:
6598:
6578:framed cobordism
6512:may be given by
6500:
6498:
6497:
6492:
6490:
6489:
6471:
6470:
6462:
6371:orthogonal group
6356:
6354:
6353:
6348:
6346:
6345:
6334:
6294:
6292:
6291:
6286:
6284:
6283:
6277:
6276:
6218:
6217:
6212:
6203:
6202:
6178:
6176:
6175:
6170:
6114:
6112:
6111:
6106:
6088:
6086:
6085:
6080:
6075:
6058:is onto for all
6057:
6055:
6054:
6049:
6044:
6039:
6031:
6030:
6022:
6021:
5998:
5996:
5995:
5990:
5982:
5978:
5974:
5966:
5965:
5964:
5963:
5950:
5932:
5924:
5923:
5922:
5921:
5908:
5885:
5883:
5882:
5877:
5875:
5867:
5866:
5848:
5847:
5831:
5829:
5828:
5823:
5818:
5813:
5805:
5804:
5799:
5798:
5775:
5773:
5772:
5767:
5752:
5750:
5749:
5744:
5742:
5741:
5732:
5728:
5727:
5726:
5688:
5687:
5661:
5659:
5658:
5653:
5651:
5615:
5613:
5612:
5607:
5605:
5598:
5593:
5581:
5580:
5575:
5574:
5557:
5552:
5541:
5536:
5524:
5523:
5518:
5517:
5493:
5492:
5487:
5486:
5469:
5464:
5452:
5451:
5446:
5445:
5421:
5420:
5415:
5414:
5397:
5392:
5380:
5379:
5374:
5373:
5341:
5339:
5338:
5333:
5331:
5327:
5323:
5315:
5314:
5309:
5295:
5294:
5267:
5265:
5264:
5259:
5257:
5256:
5251:
5238:
5236:
5235:
5230:
5228:
5224:
5223:
5222:
5217:
5200:
5199:
5163:
5161:
5160:
5155:
5153:
5149:
5148:
5147:
5142:
5125:
5124:
5103:
5102:
5083:
5081:
5080:
5075:
5073:
5072:
5067:
5058:
5054:
5032:
5031:
5030:
5029:
5003:
5002:
5001:
5000:
4978:
4974:
4952:
4951:
4950:
4949:
4923:
4922:
4921:
4920:
4891:
4889:
4888:
4883:
4875:
4874:
4856:
4855:
4839:
4837:
4836:
4831:
4823:
4822:
4784:
4782:
4781:
4776:
4774:
4770:
4769:
4768:
4750:
4749:
4728:
4726:
4725:
4720:
4715:
4714:
4709:
4708:
4658:
4656:
4655:
4650:
4642:
4641:
4619:
4617:
4616:
4611:
4609:
4608:
4589:
4587:
4586:
4581:
4579:
4575:
4568:
4567:
4540:
4535:
4534:
4520:
4519:
4514:
4505:
4504:
4499:
4498:
4477:
4475:
4474:
4469:
4467:
4466:
4461:
4460:
4404:
4402:
4401:
4396:
4394:
4393:
4388:
4387:
4351:
4349:
4348:
4343:
4341:
4340:
4335:
4334:
4326:
4308:
4307:
4302:
4301:
4284:
4282:
4281:
4276:
4224:
4222:
4221:
4216:
4214:
4213:
4208:
4195:
4193:
4192:
4187:
4185:
4184:
4179:
4178:
4164:
4162:
4161:
4156:
4101:
4099:
4098:
4093:
4047:
4045:
4044:
4041:{\displaystyle }
4039:
4021:
4019:
4018:
4013:
4011:
4010:
4005:
4004:
3990:
3988:
3987:
3982:
3922:
3920:
3919:
3914:
3911:
3908:
3906:
3890:
3888:
3887:
3882:
3880:
3879:
3874:
3873:
3828:monoidal functor
3740:quantum topology
3632:
3630:
3629:
3624:
3619:
3618:
3613:
3604:
3603:
3598:
3573:
3571:
3570:
3565:
3554:
3553:
3548:
3539:
3538:
3533:
3512:
3510:
3509:
3504:
3499:
3498:
3493:
3484:
3483:
3478:
3456:
3454:
3453:
3448:
3446:
3445:
3440:
3418:
3416:
3415:
3410:
3408:
3407:
3402:
3393:
3392:
3387:
3378:
3377:
3372:
3172:
3170:
3169:
3164:
3162:
3161:
3156:
3147:
3146:
3141:
3124:
3122:
3121:
3116:
3111:
3110:
3105:
3096:
3095:
3090:
3077:
3075:
3074:
3069:
3064:
3063:
3058:
3049:
3048:
3043:
3030:
3028:
3027:
3022:
3020:
3019:
3014:
3005:
3004:
2999:
2976:
2974:
2973:
2968:
2966:
2965:
2960:
2951:
2950:
2945:
2932:
2930:
2929:
2924:
2922:
2921:
2916:
2907:
2906:
2901:
2885:
2883:
2882:
2877:
2872:
2871:
2866:
2857:
2856:
2851:
2838:
2836:
2835:
2830:
2828:
2827:
2822:
2813:
2812:
2807:
2776:
2774:
2773:
2768:
2766:
2765:
2760:
2747:
2745:
2744:
2739:
2737:
2736:
2731:
2718:
2716:
2715:
2710:
2705:
2704:
2699:
2690:
2689:
2684:
2671:
2669:
2668:
2663:
2661:
2660:
2655:
2646:
2645:
2640:
2592:
2590:
2589:
2584:
2573:
2572:
2561:
2552:
2551:
2540:
2500:
2498:
2497:
2492:
2490:
2486:
2485:
2484:
2479:
2470:
2469:
2458:
2447:
2446:
2433:
2432:
2427:
2418:
2417:
2412:
2363:
2361:
2360:
2355:
2350:
2346:
2345:
2344:
2333:
2324:
2323:
2312:
2295:
2294:
2283:
2274:
2273:
2268:
2259:
2255:
2254:
2253:
2248:
2239:
2238:
2233:
2209:
2207:
2206:
2201:
2199:
2198:
2187:
2178:
2177:
2166:
2153:
2151:
2150:
2145:
2143:
2142:
2137:
2128:
2127:
2122:
2102:
2100:
2099:
2094:
2092:
2088:
2087:
2086:
2075:
2066:
2065:
2054:
2043:
2042:
2041:
2040:
2039:
2038:
2027:
2018:
2017:
2012:
2005:
1982:
1973:
1933:
1931:
1930:
1925:
1914:
1913:
1908:
1899:
1898:
1893:
1852:
1808:
1806:
1805:
1800:
1788:
1777:
1755:
1747:
1651:
1649:
1648:
1643:
1640:
1635:
1615:
1613:
1612:
1607:
1604:
1599:
1540:
1494:
1492:
1491:
1486:
1481:
1473:
1472:
1464:
1400:
1398:
1397:
1392:
1390:
1369:
1367:
1366:
1361:
1359:
1358:
1353:
1344:
1343:
1338:
1325:
1323:
1322:
1317:
1315:
1294:
1292:
1291:
1286:
1284:
1276:
1261:
1259:
1258:
1253:
1248:
1247:
1242:
1229:
1227:
1226:
1221:
1219:
1218:
1213:
1207:
1202:
1201:
1196:
1183:
1181:
1180:
1175:
1170:
1162:
1144:
1142:
1141:
1136:
1134:
1068:of two circles,
1036:
1029:
1022:
1008:
913:
911:
910:
905:
872:
870:
869:
864:
857:
810:
808:
807:
802:
775:
773:
772:
767:
737:
735:
734:
729:
717:
715:
714:
709:
697:
695:
694:
689:
677:
675:
674:
669:
657:
655:
654:
649:
625:
623:
622:
617:
568:
566:
565:
560:
528:
526:
525:
520:
491:
489:
488:
483:
468:
466:
465:
460:
448:
446:
445:
440:
425:
423:
422:
417:
403:
402:
390:
389:
384:
372:
371:
353:
352:
316:
314:
313:
308:
303:
302:
297:
184:
182:
181:
176:
117:piecewise linear
113:smooth manifolds
51:on the class of
9437:
9436:
9432:
9431:
9430:
9428:
9427:
9426:
9402:
9401:
9400:
9395:
9326:
9308:
9304:Urysohn's lemma
9265:
9229:
9115:
9106:
9078:low-dimensional
9036:
9031:
8987:
8961:10.2307/1970136
8921:
8895:Robert E. Stong
8857:Douglas Ravenel
8790:
8755:
8691:
8686:
8685:
8662:10.2307/1970136
8642:
8638:
8631:
8617:
8613:
8598:
8588:Springer-Verlag
8580:
8576:
8555:
8551:
8533:
8529:
8498:
8495:
8494:
8492:
8488:
8483:
8438:
8430:Stiefel numbers
8422:
8403:
8398:
8372:
8358:
8297:
8288:
8283:
8264:
8260:
8242:
8220:
8215:
8209:
8206:
8205:
8164:
8159:
8153:
8150:
8149:
8122:
8121:
8106:
8102:
8081:
8080:
8065:
8061:
8043:
8038:
8027:
8026:
8024:
8021:
8020:
8001:
7999:
7996:
7995:
7976:
7971:
7969:
7966:
7965:
7958:
7817:
7812:
7806:
7803:
7802:
7783:
7774:
7769:
7756:
7751:
7745:
7742:
7741:
7707:
7702:
7696:
7693:
7692:
7658:
7653:
7647:
7644:
7643:
7613:
7608:
7602:
7599:
7598:
7572:
7567:
7561:
7558:
7557:
7538:
7509:
7500:
7492:
7480:
7477:
7476:
7443:
7432:
7428:
7424:
7419:
7418:
7401:
7390:
7386:
7382:
7377:
7376:
7375:
7371:
7366:
7363:
7362:
7356:
7350:
7310:
7307:
7306:
7290:
7269:
7265:
7263:
7260:
7259:
7230:
7229:
7220:
7215:
7214:
7207:
7201:
7196:
7189:
7188:
7180:
7173:
7167:
7162:
7155:
7154:
7141:
7135:
7130:
7123:
7122:
7109:
7103:
7098:
7091:
7090:
7077:
7071:
7066:
7059:
7058:
7050:
7043:
7037:
7032:
7024:
7022:
7019:
7018:
6991:
6986:
6980:
6977:
6976:
6952:
6944:
6924:
6912:
6907:
6906:
6905:
6901:
6889:
6885:
6883:
6880:
6879:
6833:
6829:
6828:
6824:
6819:
6811:
6802:
6797:
6791:
6788:
6787:
6762:
6757:
6751:
6748:
6747:
6705:
6702:
6701:
6654:
6651:
6650:
6627:
6621:
6599:
6594:
6588:
6585:
6584:
6510:
6485:
6481:
6461:
6460:
6458:
6455:
6454:
6423:
6417:
6413:
6406:
6335:
6330:
6329:
6327:
6324:
6323:
6321:Euclidean space
6307:-structure (or
6301:
6279:
6278:
6260:
6256:
6229:
6228:
6213:
6208:
6207:
6198:
6197:
6190:
6189:
6187:
6184:
6183:
6122:
6119:
6118:
6094:
6091:
6090:
6071:
6063:
6060:
6059:
6040:
6035:
6023:
6017:
6016:
6015:
6007:
6004:
6003:
5970:
5959:
5955:
5951:
5946:
5945:
5928:
5917:
5913:
5909:
5904:
5903:
5902:
5898:
5893:
5890:
5889:
5871:
5862:
5858:
5843:
5839:
5837:
5834:
5833:
5814:
5809:
5800:
5794:
5793:
5792:
5784:
5781:
5780:
5761:
5758:
5757:
5737:
5733:
5716:
5712:
5696:
5692:
5680:
5676:
5674:
5671:
5670:
5647:
5630:
5627:
5626:
5603:
5602:
5594:
5589:
5582:
5576:
5570:
5569:
5568:
5565:
5564:
5553:
5548:
5537:
5532:
5525:
5519:
5513:
5512:
5511:
5508:
5507:
5494:
5488:
5482:
5481:
5480:
5477:
5476:
5465:
5460:
5453:
5447:
5441:
5440:
5439:
5436:
5435:
5422:
5416:
5410:
5409:
5408:
5405:
5404:
5393:
5388:
5381:
5375:
5369:
5368:
5367:
5363:
5361:
5358:
5357:
5319:
5310:
5305:
5304:
5303:
5299:
5290:
5286:
5284:
5281:
5280:
5252:
5247:
5246:
5244:
5241:
5240:
5218:
5213:
5212:
5205:
5201:
5195:
5191:
5177:
5174:
5173:
5143:
5138:
5137:
5130:
5126:
5120:
5116:
5098:
5094:
5092:
5089:
5088:
5068:
5063:
5062:
5025:
5021:
5020:
5016:
4996:
4992:
4991:
4987:
4986:
4982:
4945:
4941:
4940:
4936:
4916:
4912:
4911:
4907:
4906:
4902:
4900:
4897:
4896:
4870:
4866:
4851:
4847:
4845:
4842:
4841:
4818:
4814:
4794:
4791:
4790:
4764:
4760:
4745:
4741:
4740:
4736:
4734:
4731:
4730:
4710:
4704:
4703:
4702:
4676:
4673:
4672:
4671:are cobordant,
4637:
4633:
4625:
4622:
4621:
4604:
4600:
4598:
4595:
4594:
4563:
4559:
4536:
4530:
4526:
4525:
4521:
4515:
4510:
4509:
4500:
4494:
4493:
4492:
4490:
4487:
4486:
4462:
4456:
4455:
4454:
4434:
4431:
4430:
4389:
4383:
4382:
4381:
4367:
4364:
4363:
4336:
4330:
4329:
4328:
4316:
4303:
4297:
4296:
4295:
4293:
4290:
4289:
4234:
4231:
4230:
4209:
4204:
4203:
4201:
4198:
4197:
4180:
4174:
4173:
4172:
4170:
4167:
4166:
4111:
4108:
4107:
4057:
4054:
4053:
4027:
4024:
4023:
4006:
4000:
3999:
3998:
3996:
3993:
3992:
3940:
3937:
3936:
3907:
3902:
3896:
3893:
3892:
3875:
3869:
3868:
3867:
3865:
3862:
3861:
3854:
3848:
3787:
3748:
3697:homotopy theory
3662:
3644:of the triple (
3614:
3609:
3608:
3599:
3594:
3593:
3591:
3588:
3587:
3549:
3544:
3543:
3534:
3529:
3528:
3526:
3523:
3522:
3494:
3489:
3488:
3479:
3474:
3473:
3465:
3462:
3461:
3441:
3436:
3435:
3427:
3424:
3423:
3403:
3398:
3397:
3388:
3383:
3382:
3373:
3368:
3367:
3359:
3356:
3355:
3331:. The manifold
3261:
3185:
3183:Morse functions
3180:
3157:
3152:
3151:
3142:
3137:
3136:
3134:
3131:
3130:
3106:
3101:
3100:
3091:
3086:
3085:
3083:
3080:
3079:
3059:
3054:
3053:
3044:
3039:
3038:
3036:
3033:
3032:
3015:
3010:
3009:
3000:
2995:
2994:
2992:
2989:
2988:
2961:
2956:
2955:
2946:
2941:
2940:
2938:
2935:
2934:
2917:
2912:
2911:
2902:
2897:
2896:
2894:
2891:
2890:
2867:
2862:
2861:
2852:
2847:
2846:
2844:
2841:
2840:
2823:
2818:
2817:
2808:
2803:
2802:
2800:
2797:
2796:
2761:
2756:
2755:
2753:
2750:
2749:
2732:
2727:
2726:
2724:
2721:
2720:
2700:
2695:
2694:
2685:
2680:
2679:
2677:
2674:
2673:
2656:
2651:
2650:
2641:
2636:
2635:
2633:
2630:
2629:
2618:
2593:This is called
2562:
2557:
2556:
2541:
2536:
2535:
2533:
2530:
2529:
2480:
2475:
2474:
2459:
2454:
2453:
2452:
2448:
2428:
2423:
2422:
2413:
2408:
2407:
2406:
2402:
2379:
2376:
2375:
2371:of the surgery
2334:
2329:
2328:
2313:
2308:
2307:
2306:
2302:
2284:
2279:
2278:
2269:
2264:
2263:
2249:
2244:
2243:
2234:
2229:
2228:
2227:
2223:
2218:
2215:
2214:
2188:
2183:
2182:
2167:
2162:
2161:
2159:
2156:
2155:
2138:
2133:
2132:
2123:
2118:
2117:
2115:
2112:
2111:
2076:
2071:
2070:
2055:
2050:
2049:
2048:
2044:
2028:
2023:
2022:
2013:
2008:
2007:
2006:
2001:
2000:
1996:
1992:
1963:
1946:
1943:
1942:
1909:
1904:
1903:
1894:
1889:
1888:
1880:
1877:
1876:
1826:
1815:
1778:
1773:
1748:
1743:
1737:
1734:
1733:
1704:Robert E. Stong
1667:restricts to a
1636:
1631:
1625:
1622:
1621:
1600:
1595:
1589:
1586:
1585:
1563:
1500:bordism problem
1477:
1465:
1460:
1459:
1457:
1454:
1453:
1407:
1383:
1375:
1372:
1371:
1354:
1349:
1348:
1339:
1334:
1333:
1331:
1328:
1327:
1308:
1300:
1297:
1296:
1277:
1272:
1267:
1264:
1263:
1243:
1238:
1237:
1235:
1232:
1231:
1214:
1209:
1208:
1203:
1197:
1192:
1191:
1189:
1186:
1185:
1163:
1158:
1153:
1150:
1149:
1127:
1119:
1116:
1115:
1031:
1024:
1014:
1003:
997:
991:are cobordant.
982:
971:
932:cobordism class
881:
878:
877:
819:
816:
815:
781:
778:
777:
746:
743:
742:
723:
720:
719:
703:
700:
699:
683:
680:
679:
663:
660:
659:
631:
628:
627:
581:
578:
577:
542:
539:
538:
535:
505:
502:
501:
494:closed manifold
474:
471:
470:
454:
451:
450:
434:
431:
430:
398:
394:
385:
380:
379:
367:
363:
348:
344:
336:
333:
332:
298:
293:
292:
290:
287:
286:
284:Euclidean space
258:
253:
155:
152:
151:
17:
12:
11:
5:
9435:
9425:
9424:
9422:Surgery theory
9419:
9414:
9397:
9396:
9394:
9393:
9383:
9382:
9381:
9376:
9371:
9356:
9346:
9336:
9324:
9313:
9310:
9309:
9307:
9306:
9301:
9296:
9291:
9286:
9281:
9275:
9273:
9267:
9266:
9264:
9263:
9258:
9253:
9251:Winding number
9248:
9243:
9237:
9235:
9231:
9230:
9228:
9227:
9222:
9217:
9212:
9207:
9202:
9197:
9192:
9191:
9190:
9185:
9183:homotopy group
9175:
9174:
9173:
9168:
9163:
9158:
9153:
9143:
9138:
9133:
9123:
9121:
9117:
9116:
9109:
9107:
9105:
9104:
9099:
9094:
9093:
9092:
9082:
9081:
9080:
9070:
9065:
9060:
9055:
9050:
9044:
9042:
9038:
9037:
9030:
9029:
9022:
9015:
9007:
9001:
9000:
8994:
8986:
8985:External links
8983:
8982:
8981:
8943:Wall, C. T. C.
8939:
8925:
8919:
8902:
8892:
8885:Yuli B. Rudyak
8882:
8864:
8854:
8847:Daniel Quillen
8844:
8837:Lev Pontryagin
8834:
8823:Sergei Novikov
8820:
8794:
8788:
8764:
8759:
8753:
8733:
8723:
8702:
8690:
8687:
8684:
8683:
8656:(2): 292–311.
8636:
8629:
8611:
8596:
8574:
8549:
8527:
8514:
8511:
8508:
8505:
8502:
8493:The notation "
8485:
8484:
8482:
8479:
8478:
8477:
8472:
8470:Cobordism ring
8467:
8462:
8457:
8452:
8447:
8437:
8434:
8432:are the same.
8421:
8418:
8401:
8370:
8356:
8322:
8321:
8310:
8307:
8304:
8296:
8291:
8286:
8282:
8278:
8275:
8272:
8267:
8263:
8257:
8254:
8251:
8248:
8245:
8241:
8237:
8234:
8231:
8228:
8223:
8218:
8214:
8178:
8175:
8172:
8167:
8162:
8158:
8139:
8138:
8125:
8120:
8117:
8114:
8109:
8105:
8101:
8098:
8095:
8092:
8089:
8086:
8083:
8082:
8079:
8076:
8073:
8068:
8064:
8060:
8057:
8054:
8051:
8046:
8041:
8037:
8033:
8032:
8030:
8004:
7983:
7979:
7974:
7956:
7831:
7828:
7825:
7820:
7815:
7811:
7790:
7782:
7777:
7772:
7768:
7764:
7759:
7754:
7750:
7721:
7718:
7715:
7710:
7705:
7701:
7672:
7669:
7666:
7661:
7656:
7652:
7638:for any space
7627:
7624:
7621:
7616:
7611:
7607:
7586:
7583:
7580:
7575:
7570:
7566:
7537:
7534:
7512:
7508:
7498:
7495:
7491:
7487:
7484:
7473:
7472:
7461:
7458:
7454:
7450:
7446:
7442:
7435:
7431:
7427:
7422:
7417:
7414:
7411:
7408:
7404:
7400:
7393:
7389:
7385:
7380:
7374:
7370:
7354:
7348:
7326:
7323:
7320:
7317:
7314:
7293:
7289:
7286:
7283:
7280:
7275:
7272:
7268:
7244:
7243:
7228:
7223:
7218:
7213:
7210:
7208:
7199:
7195:
7191:
7190:
7187:
7183:
7179:
7176:
7174:
7165:
7161:
7157:
7156:
7153:
7150:
7147:
7144:
7142:
7133:
7129:
7125:
7124:
7121:
7118:
7115:
7112:
7110:
7101:
7097:
7093:
7092:
7089:
7086:
7083:
7080:
7078:
7069:
7065:
7061:
7060:
7057:
7053:
7049:
7046:
7044:
7035:
7031:
7027:
7026:
6989:
6985:
6969:
6968:
6950:
6947:
6943:
6939:
6935:
6931:
6927:
6923:
6918:
6915:
6910:
6904:
6900:
6895:
6892:
6888:
6873:
6872:
6861:
6857:
6853:
6850:
6847:
6844:
6839:
6836:
6832:
6827:
6822:
6818:
6814:
6810:
6800:
6796:
6770:
6760:
6756:
6724:
6721:
6718:
6715:
6712:
6709:
6673:
6670:
6667:
6664:
6661:
6658:
6620:
6617:
6602:
6597:
6593:
6566:unitary group
6508:
6488:
6484:
6480:
6477:
6474:
6468:
6465:
6421:
6415:
6411:
6404:
6344:
6341:
6338:
6333:
6300:
6297:
6296:
6295:
6282:
6275:
6272:
6269:
6266:
6263:
6259:
6255:
6252:
6249:
6246:
6243:
6240:
6237:
6234:
6231:
6230:
6227:
6224:
6221:
6216:
6211:
6206:
6201:
6196:
6195:
6193:
6168:
6165:
6162:
6159:
6156:
6153:
6150:
6147:
6144:
6141:
6138:
6135:
6132:
6129:
6126:
6104:
6101:
6098:
6078:
6074:
6070:
6067:
6047:
6043:
6038:
6034:
6029:
6026:
6020:
6014:
6011:
6000:
5999:
5988:
5985:
5981:
5977:
5973:
5969:
5962:
5958:
5954:
5949:
5944:
5941:
5938:
5935:
5931:
5927:
5920:
5916:
5912:
5907:
5901:
5897:
5874:
5870:
5865:
5861:
5857:
5854:
5851:
5846:
5842:
5821:
5817:
5812:
5808:
5803:
5797:
5791:
5788:
5765:
5754:
5753:
5740:
5736:
5731:
5725:
5722:
5719:
5715:
5711:
5708:
5705:
5702:
5699:
5695:
5691:
5686:
5683:
5679:
5650:
5646:
5643:
5640:
5637:
5634:
5617:
5616:
5601:
5597:
5592:
5588:
5585:
5583:
5579:
5573:
5567:
5566:
5563:
5560:
5556:
5551:
5547:
5544:
5540:
5535:
5531:
5528:
5526:
5522:
5516:
5510:
5509:
5506:
5503:
5500:
5497:
5495:
5491:
5485:
5479:
5478:
5475:
5472:
5468:
5463:
5459:
5456:
5454:
5450:
5444:
5438:
5437:
5434:
5431:
5428:
5425:
5423:
5419:
5413:
5407:
5406:
5403:
5400:
5396:
5391:
5387:
5384:
5382:
5378:
5372:
5366:
5365:
5330:
5326:
5322:
5318:
5313:
5308:
5302:
5298:
5293:
5289:
5255:
5250:
5227:
5221:
5216:
5211:
5208:
5204:
5198:
5194:
5190:
5187:
5184:
5181:
5152:
5146:
5141:
5136:
5133:
5129:
5123:
5119:
5115:
5112:
5109:
5106:
5101:
5097:
5085:
5084:
5071:
5066:
5061:
5057:
5053:
5050:
5047:
5044:
5041:
5038:
5035:
5028:
5024:
5019:
5015:
5012:
5009:
5006:
4999:
4995:
4990:
4985:
4981:
4977:
4973:
4970:
4967:
4964:
4961:
4958:
4955:
4948:
4944:
4939:
4935:
4932:
4929:
4926:
4919:
4915:
4910:
4905:
4881:
4878:
4873:
4869:
4865:
4862:
4859:
4854:
4850:
4829:
4826:
4821:
4817:
4813:
4810:
4807:
4804:
4801:
4798:
4773:
4767:
4763:
4759:
4756:
4753:
4748:
4744:
4739:
4718:
4713:
4707:
4701:
4698:
4695:
4692:
4689:
4686:
4683:
4680:
4648:
4645:
4640:
4636:
4632:
4629:
4607:
4603:
4591:
4590:
4578:
4574:
4571:
4566:
4562:
4558:
4555:
4552:
4549:
4546:
4543:
4539:
4533:
4529:
4524:
4518:
4513:
4508:
4503:
4497:
4465:
4459:
4453:
4450:
4447:
4444:
4441:
4438:
4423:tangent bundle
4392:
4386:
4380:
4377:
4374:
4371:
4357:graded algebra
4353:
4352:
4339:
4333:
4325:
4322:
4319:
4315:
4311:
4306:
4300:
4274:
4271:
4268:
4265:
4262:
4259:
4256:
4253:
4250:
4247:
4244:
4241:
4238:
4212:
4207:
4183:
4177:
4154:
4151:
4148:
4145:
4142:
4139:
4136:
4133:
4130:
4127:
4124:
4121:
4118:
4115:
4091:
4088:
4085:
4082:
4079:
4076:
4073:
4070:
4067:
4064:
4061:
4037:
4034:
4031:
4009:
4003:
3980:
3977:
3974:
3971:
3968:
3965:
3962:
3959:
3956:
3953:
3950:
3947:
3944:
3905:
3901:
3878:
3872:
3847:
3844:
3783:
3747:
3744:
3689:Lev Pontryagin
3674:Dieudonné 1989
3666:Henri Poincaré
3661:
3658:
3622:
3617:
3612:
3607:
3602:
3597:
3578:obtained from
3563:
3560:
3557:
3552:
3547:
3542:
3537:
3532:
3521:by surgery on
3517:obtained from
3502:
3497:
3492:
3487:
3482:
3477:
3472:
3469:
3444:
3439:
3434:
3431:
3419:between the 2-
3406:
3401:
3396:
3391:
3386:
3381:
3376:
3371:
3366:
3363:
3260:
3257:
3193:Morse function
3184:
3181:
3179:
3178:
3160:
3155:
3150:
3145:
3140:
3114:
3109:
3104:
3099:
3094:
3089:
3067:
3062:
3057:
3052:
3047:
3042:
3018:
3013:
3008:
3003:
2998:
2978:
2964:
2959:
2954:
2949:
2944:
2920:
2915:
2910:
2905:
2900:
2887:
2875:
2870:
2865:
2860:
2855:
2850:
2826:
2821:
2816:
2811:
2806:
2764:
2759:
2735:
2730:
2708:
2703:
2698:
2693:
2688:
2683:
2672:and gluing in
2659:
2654:
2649:
2644:
2639:
2617:
2614:
2582:
2579:
2576:
2571:
2568:
2565:
2560:
2555:
2550:
2547:
2544:
2539:
2528:by surgery on
2502:
2501:
2489:
2483:
2478:
2473:
2468:
2465:
2462:
2457:
2451:
2445:
2442:
2439:
2436:
2431:
2426:
2421:
2416:
2411:
2405:
2401:
2398:
2395:
2392:
2389:
2386:
2383:
2365:
2364:
2353:
2349:
2343:
2340:
2337:
2332:
2327:
2322:
2319:
2316:
2311:
2305:
2301:
2298:
2293:
2290:
2287:
2282:
2277:
2272:
2267:
2262:
2258:
2252:
2247:
2242:
2237:
2232:
2226:
2222:
2197:
2194:
2191:
2186:
2181:
2176:
2173:
2170:
2165:
2154:and gluing in
2141:
2136:
2131:
2126:
2121:
2104:
2103:
2091:
2085:
2082:
2079:
2074:
2069:
2064:
2061:
2058:
2053:
2047:
2037:
2034:
2031:
2026:
2021:
2016:
2011:
2004:
1999:
1995:
1991:
1988:
1985:
1981:
1978:
1972:
1969:
1966:
1962:
1959:
1956:
1953:
1950:
1923:
1920:
1917:
1912:
1907:
1902:
1897:
1892:
1887:
1884:
1814:
1811:
1798:
1795:
1792:
1787:
1784:
1781:
1776:
1772:
1768:
1765:
1762:
1759:
1754:
1751:
1746:
1742:
1713:is surgery on
1711:surgery theory
1671:-structure on
1663:-structure on
1639:
1634:
1630:
1603:
1598:
1594:
1583:cobordism ring
1562:
1559:
1484:
1480:
1476:
1471:
1468:
1463:
1419:null-cobordant
1406:
1403:
1389:
1386:
1382:
1379:
1357:
1352:
1347:
1342:
1337:
1314:
1311:
1307:
1304:
1283:
1280:
1275:
1271:
1251:
1246:
1241:
1217:
1212:
1206:
1200:
1195:
1173:
1169:
1166:
1161:
1157:
1133:
1130:
1126:
1123:
1060:consists of a
996:
993:
980:
976: = ∂
969:
965: = ∂
903:
900:
897:
894:
891:
888:
885:
874:
873:
862:
856:
853:
850:
847:
844:
841:
838:
835:
832:
829:
826:
823:
800:
797:
794:
791:
788:
785:
765:
762:
759:
756:
753:
750:
727:
707:
687:
667:
647:
644:
641:
638:
635:
615:
612:
609:
606:
603:
600:
597:
594:
591:
588:
585:
558:
555:
552:
549:
546:
534:
531:
518:
515:
512:
509:
492:. Finally, a
481:
478:
469:is denoted by
458:
438:
427:
426:
415:
412:
409:
406:
401:
397:
393:
388:
383:
378:
375:
370:
366:
362:
359:
356:
351:
347:
343:
340:
306:
301:
296:
257:
254:
252:
249:
233:surgery theory
198:diffeomorphism
190:diffeomorphism
174:
171:
168:
165:
162:
159:
78:disjoint union
15:
9:
6:
4:
3:
2:
9434:
9423:
9420:
9418:
9415:
9413:
9410:
9409:
9407:
9392:
9384:
9380:
9377:
9375:
9372:
9370:
9367:
9366:
9365:
9357:
9355:
9351:
9347:
9345:
9341:
9337:
9335:
9330:
9325:
9323:
9315:
9314:
9311:
9305:
9302:
9300:
9297:
9295:
9292:
9290:
9287:
9285:
9282:
9280:
9277:
9276:
9274:
9272:
9268:
9262:
9261:Orientability
9259:
9257:
9254:
9252:
9249:
9247:
9244:
9242:
9239:
9238:
9236:
9232:
9226:
9223:
9221:
9218:
9216:
9213:
9211:
9208:
9206:
9203:
9201:
9198:
9196:
9193:
9189:
9186:
9184:
9181:
9180:
9179:
9176:
9172:
9169:
9167:
9164:
9162:
9159:
9157:
9154:
9152:
9149:
9148:
9147:
9144:
9142:
9139:
9137:
9134:
9132:
9128:
9125:
9124:
9122:
9118:
9113:
9103:
9100:
9098:
9097:Set-theoretic
9095:
9091:
9088:
9087:
9086:
9083:
9079:
9076:
9075:
9074:
9071:
9069:
9066:
9064:
9061:
9059:
9058:Combinatorial
9056:
9054:
9051:
9049:
9046:
9045:
9043:
9039:
9035:
9028:
9023:
9021:
9016:
9014:
9009:
9008:
9005:
8998:
8995:
8992:
8989:
8988:
8978:
8974:
8970:
8966:
8962:
8958:
8954:
8950:
8949:
8944:
8940:
8937:
8933:
8929:
8926:
8922:
8916:
8912:
8908:
8903:
8900:
8896:
8893:
8890:
8886:
8883:
8880:
8876:
8875:
8870:
8865:
8862:
8858:
8855:
8852:
8848:
8845:
8842:
8838:
8835:
8832:
8828:
8824:
8821:
8817:
8813:
8809:
8805:
8804:
8799:
8795:
8791:
8785:
8781:
8777:
8773:
8769:
8765:
8760:
8756:
8750:
8745:
8744:
8738:
8734:
8731:
8727:
8724:
8721:
8717:
8716:
8711:
8707:
8703:
8700:
8696:
8693:
8692:
8679:
8675:
8671:
8667:
8663:
8659:
8655:
8651:
8647:
8640:
8632:
8630:0-12-583430-6
8626:
8622:
8615:
8607:
8603:
8599:
8593:
8589:
8585:
8578:
8571:
8567:
8563:
8559:
8553:
8545:
8541:
8537:
8531:
8509:
8506:
8503:
8490:
8486:
8476:
8473:
8471:
8468:
8466:
8463:
8461:
8458:
8456:
8453:
8451:
8448:
8446:
8444:
8440:
8439:
8433:
8431:
8427:
8420:Other results
8417:
8415:
8411:
8407:
8396:
8392:
8388:
8383:
8381:
8377:
8373:
8367:
8363:
8359:
8353:
8349:
8345:
8342:
8337:
8335:
8331:
8327:
8308:
8289:
8284:
8276:
8273:
8265:
8261:
8255:
8252:
8249:
8246:
8243:
8239:
8235:
8229:
8221:
8216:
8204:
8203:
8202:
8200:
8196:
8193:, though the
8192:
8173:
8165:
8160:
8147:
8142:
8115:
8107:
8103:
8093:
8090:
8087:
8074:
8066:
8062:
8052:
8044:
8039:
8028:
8019:
8018:
8017:
7981:
7977:
7963:
7959:
7952:
7948:
7944:
7939:
7937:
7933:
7929:
7925:
7921:
7917:
7913:
7910:) with a map
7909:
7905:
7901:
7897:
7893:
7889:
7885:
7881:
7877:
7873:
7869:
7865:
7861:
7857:
7853:
7849:
7845:
7826:
7818:
7813:
7775:
7770:
7762:
7757:
7752:
7739:
7735:
7734:contravariant
7716:
7708:
7703:
7690:
7686:
7667:
7659:
7654:
7641:
7622:
7614:
7609:
7581:
7573:
7568:
7555:
7551:
7547:
7543:
7542:vector bundle
7533:
7531:
7527:
7496:
7493:
7485:
7482:
7459:
7456:
7452:
7433:
7429:
7425:
7415:
7412:
7409:
7391:
7387:
7383:
7372:
7368:
7361:
7360:
7359:
7357:
7347:
7342:
7340:
7324:
7318:
7312:
7287:
7281:
7273:
7270:
7266:
7257:
7253:
7249:
7226:
7221:
7211:
7209:
7197:
7185:
7177:
7175:
7163:
7151:
7148:
7145:
7143:
7131:
7119:
7116:
7113:
7111:
7099:
7087:
7084:
7081:
7079:
7067:
7055:
7047:
7045:
7033:
7017:
7016:
7015:
7012:
7010:
6987:
6974:
6948:
6945:
6937:
6933:
6916:
6913:
6902:
6898:
6893:
6890:
6886:
6878:
6877:
6876:
6859:
6855:
6851:
6848:
6845:
6842:
6837:
6834:
6830:
6825:
6816:
6808:
6798:
6786:
6785:
6784:
6781:
6768:
6758:
6745:
6740:
6738:
6719:
6716:
6710:
6707:
6699:
6696: ×
6695:
6691:
6687:
6668:
6665:
6659:
6656:
6648:
6644:
6640:
6636:
6632:
6626:
6616:
6600:
6595:
6581:
6579:
6575:
6573:
6569:
6563:
6559:
6555:
6553:
6547:
6543:
6539:
6535:
6531:
6527:
6523:
6519:
6515:
6511:
6504:
6486:
6482:
6475:
6472:
6463:
6452:
6448:
6444:
6440:
6436:
6432:
6428:
6424:
6414:
6407:
6400:
6396:
6392:
6389: +
6388:
6384:
6380:
6376:
6372:
6368:
6364:
6360:
6342:
6339:
6336:
6322:
6318:
6314:
6313:normal bundle
6310:
6306:
6270:
6264:
6261:
6257:
6250:
6244:
6235:
6222:
6214:
6191:
6182:
6181:
6180:
6163:
6157:
6151:
6145:
6142:
6136:
6133:
6130:
6124:
6115:
6102:
6099:
6096:
6076:
6068:
6065:
6045:
6041:
6027:
6024:
6012:
6009:
5986:
5983:
5979:
5960:
5956:
5952:
5942:
5939:
5936:
5918:
5914:
5910:
5899:
5895:
5888:
5887:
5886:
5868:
5863:
5859:
5855:
5852:
5849:
5844:
5840:
5819:
5815:
5801:
5789:
5786:
5777:
5763:
5738:
5734:
5729:
5723:
5720:
5717:
5709:
5706:
5700:
5697:
5693:
5689:
5684:
5677:
5669:
5668:
5667:
5665:
5644:
5638:
5632:
5625:
5620:
5599:
5595:
5586:
5584:
5577:
5561:
5558:
5554:
5545:
5542:
5538:
5529:
5527:
5520:
5504:
5501:
5498:
5496:
5489:
5473:
5470:
5466:
5457:
5455:
5448:
5432:
5429:
5426:
5424:
5417:
5401:
5398:
5394:
5385:
5383:
5376:
5356:
5355:
5354:
5351:
5349:
5346:-dimensional
5345:
5328:
5311:
5300:
5296:
5291:
5287:
5278:
5273:
5271:
5268:-coefficient
5253:
5225:
5219:
5209:
5206:
5202:
5196:
5192:
5188:
5182:
5171:
5167:
5150:
5144:
5134:
5131:
5127:
5121:
5117:
5113:
5107:
5099:
5095:
5069:
5059:
5055:
5048:
5042:
5036:
5026:
5022:
5017:
5013:
5007:
4997:
4993:
4988:
4983:
4979:
4975:
4968:
4962:
4956:
4946:
4942:
4937:
4933:
4927:
4917:
4913:
4908:
4903:
4895:
4894:
4893:
4879:
4876:
4871:
4867:
4863:
4860:
4857:
4852:
4848:
4827:
4824:
4819:
4815:
4811:
4808:
4805:
4802:
4799:
4796:
4788:
4771:
4765:
4761:
4757:
4754:
4751:
4746:
4742:
4737:
4716:
4711:
4699:
4693:
4687:
4681:
4670:
4666:
4662:
4646:
4643:
4638:
4634:
4630:
4627:
4605:
4601:
4576:
4572:
4569:
4564:
4560:
4556:
4553:
4550:
4547:
4544:
4541:
4531:
4527:
4522:
4516:
4506:
4501:
4485:
4484:
4483:
4481:
4463:
4451:
4448:
4445:
4439:
4428:
4424:
4420:
4416:
4412:
4408:
4390:
4378:
4372:
4360:
4358:
4337:
4323:
4320:
4317:
4313:
4309:
4304:
4288:
4287:
4286:
4272:
4266:
4263:
4260:
4254:
4248:
4239:
4228:
4210:
4181:
4165:. Therefore,
4146:
4143:
4140:
4134:
4131:
4122:
4119:
4116:
4113:
4105:
4080:
4074:
4068:
4062:
4051:
4007:
3975:
3972:
3969:
3963:
3957:
3951:
3945:
3934:
3930:
3926:
3925:abelian group
3903:
3876:
3859:
3853:
3843:
3839:
3837:
3833:
3832:vector spaces
3829:
3825:
3820:
3818:
3814:
3810:
3806:
3802:
3798:
3794:
3790:
3786:
3781:
3777:
3773:
3769:
3765:
3761:
3757:
3753:
3743:
3741:
3737:
3733:
3729:
3725:
3720:
3718:
3714:
3710:
3706:
3702:
3698:
3694:
3690:
3685:
3683:
3679:
3675:
3671:
3667:
3657:
3655:
3651:
3647:
3643:
3639:
3620:
3615:
3605:
3600:
3585:
3581:
3577:
3561:
3558:
3555:
3550:
3540:
3535:
3520:
3516:
3500:
3495:
3485:
3480:
3470:
3467:
3460:
3442:
3432:
3429:
3422:
3404:
3394:
3389:
3379:
3374:
3364:
3361:
3352:
3348:
3346:
3342:
3338:
3334:
3330:
3326:
3322:
3318:
3314:
3310:
3306:
3302:
3298:
3294:
3290:
3286:
3283:→ such that
3282:
3278:
3274:
3270:
3266:
3256:
3254:
3250:
3246:
3242:
3238:
3234:
3230:
3226:
3222:
3218:
3214:
3210:
3206:
3202:
3198:
3194:
3190:
3187:Suppose that
3176:
3158:
3148:
3143:
3129:
3112:
3107:
3097:
3092:
3065:
3060:
3050:
3045:
3016:
3006:
3001:
2983:
2979:
2962:
2952:
2947:
2918:
2908:
2903:
2889:
2888:
2886:
2873:
2868:
2858:
2853:
2824:
2814:
2809:
2789:
2781:
2777:
2762:
2733:
2706:
2701:
2691:
2686:
2657:
2647:
2642:
2622:
2613:
2611:
2607:
2603:
2602:Marston Morse
2598:
2596:
2580:
2577:
2574:
2569:
2566:
2563:
2553:
2548:
2545:
2542:
2527:
2523:
2520:). Note that
2519:
2515:
2511:
2507:
2487:
2481:
2471:
2466:
2463:
2460:
2449:
2440:
2434:
2429:
2419:
2414:
2403:
2396:
2393:
2390:
2384:
2381:
2374:
2373:
2372:
2370:
2351:
2347:
2341:
2338:
2335:
2325:
2320:
2317:
2314:
2303:
2296:
2291:
2288:
2285:
2275:
2270:
2260:
2256:
2250:
2240:
2235:
2224:
2213:
2212:
2211:
2195:
2192:
2189:
2179:
2174:
2171:
2168:
2139:
2129:
2124:
2109:
2089:
2083:
2080:
2077:
2067:
2062:
2059:
2056:
2045:
2035:
2032:
2029:
2019:
2014:
1997:
1993:
1986:
1983:
1960:
1957:
1951:
1948:
1941:
1940:
1939:
1937:
1921:
1918:
1915:
1910:
1900:
1895:
1885:
1882:
1875:
1871:
1867:
1863:
1860:of dimension
1859:
1854:
1850:
1846:
1842:
1838:
1834:
1830:
1824:
1820:
1810:
1793:
1785:
1782:
1779:
1774:
1766:
1760:
1752:
1749:
1744:
1731:
1727:
1722:
1720:
1716:
1712:
1707:
1705:
1701:
1698:
1694:
1690:
1686:
1682:
1678:
1674:
1670:
1666:
1662:
1657:
1655:
1637:
1632:
1619:
1601:
1596:
1584:
1580:
1576:
1572:
1568:
1558:
1556:
1552:
1548:
1544:
1539:
1538:
1532:
1527:
1525:
1521:
1516:
1512:
1508:
1503:
1501:
1496:
1469:
1466:
1452:
1449:-dimensional
1448:
1444:
1440:
1436:
1432:
1428:
1424:
1420:
1416:
1412:
1402:
1387:
1384:
1380:
1377:
1355:
1345:
1340:
1312:
1309:
1305:
1302:
1281:
1278:
1269:
1249:
1244:
1215:
1198:
1171:
1167:
1164:
1155:
1148:
1147:connected sum
1131:
1128:
1124:
1121:
1113:
1109:
1105:
1100:
1098:
1094:
1090:
1086:
1082:
1079:
1078:pair of pants
1075:
1071:
1067:
1063:
1059:
1050:
1046:
1044:
1040:
1034:
1027:
1021:
1017:
1012:
1006:
1002:
1001:unit interval
992:
990:
986:
979:
975:
968:
964:
960:
956:
952:
948:
944:
939:
937:
933:
929:
925:
921:
917:
898:
895:
892:
889:
886:
860:
851:
845:
842:
836:
830:
827:
824:
814:
813:
812:
798:
789:
786:
783:
763:
754:
751:
748:
741:
725:
705:
685:
665:
642:
639:
636:
610:
607:
604:
601:
598:
595:
592:
589:
586:
576:
572:
569:-dimensional
553:
550:
547:
530:
513:
510:
499:
495:
479:
456:
436:
413:
407:
404:
399:
395:
391:
386:
376:
368:
364:
360:
357:
354:
349:
345:
331:
330:
329:
328:
324:
320:
304:
299:
285:
281:
277:
274:
270:
267:
264:-dimensional
263:
248:
246:
242:
238:
234:
230:
228:
223:
219:
215:
211:
207:
203:
202:homeomorphism
199:
195:
194:homeomorphism
191:
186:
172:
169:
166:
163:
160:
149:
145:
141:
137:
133:
129:
124:
122:
118:
114:
110:
105:
101:
97:
94:
90:
85:
83:
79:
75:
71:
67:
66:
61:
57:
54:
50:
46:
42:
34:
30:
26:
23:A cobordism (
21:
9391:Publications
9256:Chern number
9246:Betti number
9224:
9129: /
9120:Key concepts
9068:Differential
8952:
8946:
8931:
8906:
8898:
8888:
8872:
8860:
8850:
8840:
8830:
8826:
8807:
8801:
8798:Milnor, John
8771:
8742:
8729:
8713:
8698:
8653:
8649:
8639:
8620:
8614:
8609:, chapter 12
8583:
8577:
8569:
8565:
8561:
8557:
8552:
8539:
8530:
8489:
8442:
8423:
8413:
8409:
8405:
8394:
8390:
8384:
8379:
8375:
8368:
8354:
8347:
8343:
8341:Thom spectra
8338:
8323:
8190:
8143:
8140:
7961:
7954:
7946:
7942:
7940:
7935:
7931:
7927:
7923:
7919:
7915:
7911:
7907:
7903:
7899:
7895:
7891:
7887:
7883:
7879:
7875:
7871:
7867:
7863:
7859:
7855:
7851:
7847:
7843:
7737:
7688:
7639:
7539:
7529:
7525:
7474:
7352:
7345:
7343:
7255:
7251:
7245:
7013:
6970:
6874:
6782:
6743:
6741:
6697:
6693:
6689:
6685:
6646:
6642:
6638:
6634:
6628:
6582:
6571:
6567:
6551:
6545:
6541:
6533:
6529:
6525:
6521:
6517:
6513:
6506:
6502:
6450:
6446:
6442:
6438:
6434:
6430:
6426:
6419:
6409:
6402:
6398:
6394:
6390:
6386:
6382:
6378:
6374:
6363:Grassmannian
6358:
6316:
6304:
6302:
6116:
6001:
5778:
5755:
5663:
5621:
5618:
5352:
5343:
5276:
5274:
5165:
5086:
4786:
4668:
4664:
4660:
4592:
4426:
4418:
4410:
4406:
4361:
4354:
4103:
4049:
3932:
3928:
3923:); it is an
3857:
3855:
3840:
3821:
3812:
3808:
3804:
3796:
3792:
3788:
3784:
3779:
3775:
3771:
3767:
3763:
3759:
3755:
3749:
3721:
3707:, alongside
3701:Thom complex
3686:
3663:
3653:
3649:
3645:
3637:
3635:
3583:
3579:
3575:
3518:
3514:
3344:
3336:
3332:
3328:
3324:
3320:
3316:
3312:
3308:
3304:
3300:
3296:
3292:
3288:
3284:
3280:
3276:
3272:
3268:
3264:
3262:
3252:
3248:
3244:
3240:
3236:
3232:
3228:
3224:
3220:
3216:
3212:
3208:
3204:
3200:
3196:
3188:
3186:
3175:Klein bottle
2794:
2627:
2599:
2594:
2525:
2521:
2517:
2513:
2509:
2505:
2503:
2368:
2366:
2106:obtained by
2105:
1935:
1869:
1865:
1861:
1857:
1855:
1848:
1844:
1840:
1836:
1832:
1828:
1822:
1818:
1816:
1723:
1708:
1696:
1688:
1684:
1680:
1676:
1672:
1668:
1664:
1660:
1658:
1582:
1564:
1554:
1550:
1546:
1542:
1530:
1528:
1523:
1519:
1514:
1510:
1504:
1499:
1498:The general
1497:
1446:
1438:
1434:
1430:
1426:
1422:
1418:
1414:
1410:
1408:
1111:
1107:
1103:
1101:
1096:
1092:
1088:
1084:
1080:
1073:
1069:
1065:
1057:
1055:
1042:
1038:
1032:
1025:
1019:
1015:
1010:
1004:
998:
988:
984:
977:
973:
966:
962:
958:
954:
950:
946:
942:
940:
935:
931:
927:
923:
919:
915:
875:
698:-manifolds
570:
536:
428:
322:
280:homeomorphic
268:
261:
259:
226:
222:Morse theory
187:
147:
143:
139:
135:
131:
127:
125:
103:
99:
95:
88:
86:
81:
73:
69:
63:
44:
38:
32:
28:
24:
9354:Wikiversity
9271:Key results
8869:"Cobordism"
8352:Thom spaces
6538:G-structure
6309:G-structure
5779:Therefore,
2610:John Milnor
2508:cobordism (
2504:defines an
1934:define the
1715:normal maps
1581:called the
1579:graded ring
1571:G-structure
1405:Terminology
922:are called
229:-cobordisms
41:mathematics
9406:Categories
9200:CW complex
9141:Continuity
9131:Closed set
9090:cohomology
8911:S. Novikov
8768:Madsen, Ib
8689:References
8445:-cobordism
6562:spin group
6418:with maps
4840:such that
4478:. In 1954
4425:. Thus if
4102:for every
3782: ′ ∪
3770: ′;
3699:, via the
3457:and the 2-
3177:(Fig. 2c).
2506:elementary
1938:-manifold
1443:handlebody
1417:is called
1413:-manifold
740:embeddings
678:; closed
533:Cobordisms
327:half-space
251:Definition
9379:geometric
9374:algebraic
9225:Cobordism
9161:Hausdorff
9156:connected
9073:Geometric
9063:Continuum
9053:Algebraic
8997:B-Bordism
8969:0003-486X
8928:René Thom
8879:EMS Press
8816:0013-8584
8810:: 16–23.
8720:EMS Press
8710:"bordism"
8670:0003-486X
8364:over the
8281:Ω
8240:∑
8213:Ω
8157:Ω
8108:∗
8100:↦
8059:→
8036:Ω
7930:, and to
7858:a closed
7810:Ω
7767:Ω
7749:Ω
7709:∗
7700:Ω
7685:covariant
7655:∗
7651:Ω
7606:Ω
7565:Ω
7507:→
7490:Ω
7483:σ
7416:×
7413:⋯
7410:×
7369:σ
7313:σ
7288:∈
7248:signature
7194:Ω
7160:Ω
7128:Ω
7096:Ω
7064:Ω
7030:Ω
6988:∗
6984:Ω
6942:Ω
6938:∈
6849:⩾
6843:∣
6809:⊗
6799:∗
6795:Ω
6759:∗
6755:Ω
6717:−
6711:⊔
6684:, where −
6666:−
6660:⊔
6596:∗
6592:Ω
6520:), where
6479:→
6467:~
6464:ν
6265:
6245:χ
6242:↦
6205:→
6158:χ
6146:χ
6134:×
6125:χ
6069:∈
6033:→
6010:χ
5943:×
5940:⋯
5937:×
5896:χ
5869:∈
5853:⋯
5807:→
5787:χ
5735:χ
5721:
5707:−
5701:−
5682:∂
5678:χ
5645:∈
5633:χ
5546:⊕
5275:For even
5189:∈
5114:∈
5060:∈
5014:⋯
4934:⋯
4861:⋯
4825:−
4812:≠
4800:⩾
4755:⋯
4700:∈
4644:−
4631:≠
4570:−
4557:≠
4545:⩾
4502:∗
4480:René Thom
4452:∈
4379:∈
4321:⩾
4314:⨁
4305:∗
4264:×
4135:×
4126:∂
4117:⊔
4087:∅
4033:∅
3973:⊔
3900:Ω
3732:morphisms
3693:René Thom
3606:×
3556:⊂
3541:×
3486:×
3395:−
3380:×
3239: :=
3223: :=
3211: :=
3149:×
3098:×
3051:×
3007:×
2953:×
2909:×
2859:×
2815:×
2692:×
2648:×
2606:René Thom
2575:⊂
2567:−
2554:×
2472:×
2435:×
2420:×
2404:∪
2394:×
2339:−
2326:×
2300:∂
2289:−
2276:×
2241:×
2221:∂
2193:−
2180:×
2130:×
2081:−
2068:×
2033:−
2020:×
1998:φ
1994:∪
1987:φ
1984:
1961:−
1916:⊂
1901:×
1883:φ
1874:embedding
1775:∗
1771:Ω
1745:∗
1741:Ω
1633:∗
1629:Ω
1597:∗
1593:Ω
1543:Cobordism
1529:The term
1515:cobordism
1381:⊔
1346:×
1306:⊔
1274:#
1205:#
1160:#
1125:⊔
1041:× {0} to
930:form the
924:cobordant
843:⊔
822:∂
796:∂
793:↪
787::
761:∂
758:↪
752::
575:quintuple
571:cobordism
517:∅
508:∂
477:∂
405:⩾
392:∣
377:∈
358:…
256:Manifolds
170:⊔
158:∂
128:cobordism
109:René Thom
76:if their
74:cobordant
70:cobordism
68:, giving
56:manifolds
45:cobordism
9344:Wikibook
9322:Category
9210:Manifold
9178:Homotopy
9136:Interior
9127:Open set
9085:Homology
9034:Topology
8739:(1989).
8538:(1968).
8436:See also
7914: :
7870: :
7550:K-theory
6688:denotes
6631:oriented
6449:+1), an
5056:⟩
4984:⟨
4976:⟩
4904:⟨
3752:category
3724:category
3709:K-theory
3670:homology
3279: :
2616:Examples
1691:= U for
1567:oriented
1561:Variants
1507:fillings
1388:′
1313:′
1282:′
1168:′
1132:′
995:Examples
934:of
266:manifold
93:manifold
82:boundary
62:(French
60:boundary
9369:general
9171:uniform
9151:compact
9102:Digital
8991:Bordism
8977:1970136
8678:1970136
8606:1886843
8360:of the
7896:bordant
7854:) with
7844:bordism
7801:. Then
7548:called
7351:, ...,
6971:of the
6369:of the
6361:to the
4482:proved
3836:functor
3766:) and (
3728:objects
3660:History
3195:on an (
2791:Fig. 2b
2783:Fig. 2a
2108:surgery
1872:and an
1721:class.
1719:bordism
1531:bordism
1511:Bordism
1045:× {1}.
983:, then
498:compact
276:locally
80:is the
53:compact
9364:Topics
9166:metric
9041:Fields
8975:
8967:
8917:
8814:
8786:
8751:
8676:
8668:
8627:
8604:
8594:
7949:has a
7894:) are
7540:Every
6564:, the
6560:, the
4225:, the
4106:since
3801:cospan
3678:p. 289
3421:sphere
3341:handle
3295:(1) =
3287:(0) =
2624:Fig. 1
1974:
1835:) = (∂
1697:stably
1695:using
1064:, and
1062:circle
1035:× {1}
1028:× {0}
858:
738:; and
239:, and
224:, and
98:is an
9146:Space
8973:JSTOR
8674:JSTOR
8481:Notes
7532:= 1.
6373:: ν:
5087:with
4355:is a
3826:is a
3513:with
3459:torus
3191:is a
3128:torus
2369:trace
1843:) ∪ (
573:is a
271:is a
220:with
8965:ISSN
8915:ISBN
8812:ISSN
8784:ISBN
8749:ISBN
8666:ISSN
8625:ISBN
8592:ISBN
8564:and
8428:and
8378:and
7886:), (
7732:are
7683:are
7358:≥ 1
7246:The
6528:) →
6441:) →
6393:) →
5622:The
5239:the
5172:and
5164:the
3931:and
3574:and
2608:and
2367:The
1728:and
1675:and
1549:and
1537:bord
1513:and
1095:and
1087:and
1072:and
987:and
972:and
918:and
212:and
146:and
134:and
119:and
111:for
65:bord
8957:doi
8658:doi
8558:not
8410:MSO
8376:MSO
8336:).
7941:An
7934:on
7926:on
7736:in
7687:in
6700:is
6550:SO(
6262:dim
5718:dim
5168:th
4785:of
4417:of
4285:so
2839:or
1847:× ∂
1409:An
1370:in
1056:If
1013:, (
959:not
957:is
537:An
529:.)
200:or
192:or
39:In
9408::
8971:.
8963:.
8953:72
8934:,
8930:,
8897:,
8887:,
8877:,
8871:,
8859:,
8849:,
8839:,
8831:31
8825:,
8806:.
8782:.
8778::
8774:.
8728:,
8718:,
8712:,
8697:,
8672:.
8664:.
8654:72
8652:.
8648:.
8602:MR
8600:,
8590:,
8416:.
8414:MO
8406:MO
8393:=
8391:MO
8389:–
8380:MO
8369:BG
8355:MG
8344:MG
8299:pt
7953:∈
7938:.
7918:→
7906:,
7902:;
7890:,
7882:,
7874:→
7850:,
7785:pt
7502:SO
7460:1.
7341:.
7203:SO
7169:SO
7137:SO
7105:SO
7073:SO
7039:SO
6993:SO
6954:SO
6804:SO
6764:SO
6739:.
6641:,
6637:,
6615:.
6580:.
6544:=
6514:BG
6443:BO
6435:BO
6427:BO
6425:→
6416:+1
6408:→
6395:BO
6385:,
6379:Gr
6377:→
6103:1.
5987:1.
5776:.
5600:2.
5350:.
5272:.
4667:,
3822:A
3819:.
3811:←
3807:→
3803::
3795:,
3791:;
3774:,
3762:,
3758:;
3742:.
3719:.
3676:,
3652:,
3648:;
3582:×
3347:.
3319:,
3315:;
3291:,
3271:,
3267:;
3251:,
3247:;
2612:.
2604:,
2597:.
2516:,
2512:;
2385::=
1952::=
1868:+
1864:=
1853:.
1839:×
1831:×
1827:∂(
1821:,
1706:.
1656:.
1526:.
1509:.
1110:,
1030:,
1023:;
1018:×
1007:=
938:.
914:.
776:,
718:,
317:A
247:.
185:.
150:,
126:A
123:.
43:,
35:).
31:,
27:;
9026:e
9019:t
9012:v
8979:.
8959::
8923:.
8818:.
8808:8
8792:.
8757:.
8680:.
8660::
8633:.
8570:W
8566:N
8562:M
8546:.
8513:)
8510:1
8507:+
8504:n
8501:(
8443:h
8404:(
8402:∗
8399:π
8397:(
8395:H
8371:n
8357:n
8348:G
8309:.
8306:)
8303:)
8295:(
8290:G
8285:q
8277:;
8274:X
8271:(
8266:p
8262:H
8256:n
8253:=
8250:q
8247:+
8244:p
8236:=
8233:)
8230:X
8227:(
8222:G
8217:n
8191:X
8177:)
8174:X
8171:(
8166:n
8161:G
8119:]
8116:M
8113:[
8104:f
8097:)
8094:f
8091:,
8088:M
8085:(
8078:)
8075:X
8072:(
8067:n
8063:H
8056:)
8053:X
8050:(
8045:G
8040:n
8029:{
8003:Z
7982:2
7978:/
7973:Z
7962:M
7960:(
7957:n
7955:H
7947:M
7943:n
7936:N
7932:g
7928:M
7924:f
7920:X
7916:W
7912:h
7908:N
7904:M
7900:W
7892:g
7888:N
7884:f
7880:M
7876:X
7872:M
7868:f
7864:M
7860:n
7856:M
7852:f
7848:M
7830:)
7827:X
7824:(
7819:G
7814:n
7789:)
7781:(
7776:G
7771:n
7763:=
7758:G
7753:n
7738:X
7720:)
7717:X
7714:(
7704:G
7689:X
7671:)
7668:X
7665:(
7660:G
7640:X
7626:)
7623:X
7620:(
7615:n
7610:G
7585:)
7582:X
7579:(
7574:G
7569:n
7530:i
7526:i
7511:Z
7497:i
7494:4
7486::
7457:=
7453:)
7449:)
7445:C
7441:(
7434:k
7430:i
7426:2
7421:P
7407:)
7403:C
7399:(
7392:1
7388:i
7384:2
7379:P
7373:(
7355:k
7353:i
7349:1
7346:i
7325:.
7322:)
7319:M
7316:(
7292:Z
7285:)
7282:M
7279:(
7274:i
7271:2
7267:H
7256:M
7252:i
7227:.
7222:2
7217:Z
7212:=
7198:5
7186:,
7182:Z
7178:=
7164:4
7152:,
7149:0
7146:=
7132:3
7120:,
7117:0
7114:=
7100:2
7088:,
7085:0
7082:=
7068:1
7056:,
7052:Z
7048:=
7034:0
6949:i
6946:4
6934:]
6930:)
6926:C
6922:(
6917:i
6914:2
6909:P
6903:[
6899:=
6894:i
6891:4
6887:y
6860:,
6856:]
6852:1
6846:i
6838:i
6835:4
6831:y
6826:[
6821:Q
6817:=
6813:Q
6769:.
6744:M
6723:)
6720:M
6714:(
6708:M
6698:I
6694:M
6690:N
6686:N
6672:)
6669:N
6663:(
6657:M
6643:N
6639:M
6635:W
6601:G
6574:)
6572:k
6570:(
6568:U
6554:)
6552:k
6546:O
6542:G
6534:k
6532:(
6530:O
6526:k
6524:(
6522:G
6518:k
6516:(
6509:k
6507:X
6503:X
6487:k
6483:X
6476:M
6473::
6451:X
6447:k
6445:(
6439:k
6437:(
6431:k
6429:(
6422:k
6420:X
6412:k
6410:X
6405:k
6403:X
6399:k
6397:(
6391:k
6387:n
6383:n
6381:(
6375:M
6359:M
6343:k
6340:+
6337:n
6332:R
6317:M
6305:X
6274:)
6271:M
6268:(
6258:x
6254:)
6251:M
6248:(
6239:]
6236:M
6233:[
6226:]
6223:x
6220:[
6215:2
6210:F
6200:N
6192:{
6167:)
6164:N
6161:(
6155:)
6152:M
6149:(
6143:=
6140:)
6137:N
6131:M
6128:(
6100:=
6097:i
6077:,
6073:N
6066:i
6046:2
6042:/
6037:Z
6028:i
6025:2
6019:N
6013::
5984:=
5980:)
5976:)
5972:R
5968:(
5961:k
5957:i
5953:2
5948:P
5934:)
5930:R
5926:(
5919:1
5915:i
5911:2
5906:P
5900:(
5873:N
5864:k
5860:i
5856:,
5850:,
5845:1
5841:i
5820:2
5816:/
5811:Z
5802:i
5796:N
5790::
5764:W
5739:W
5730:)
5724:W
5714:)
5710:1
5704:(
5698:1
5694:(
5690:=
5685:W
5664:M
5649:Z
5642:)
5639:M
5636:(
5596:/
5591:Z
5587:=
5578:5
5572:N
5562:,
5559:2
5555:/
5550:Z
5543:2
5539:/
5534:Z
5530:=
5521:4
5515:N
5505:,
5502:0
5499:=
5490:3
5484:N
5474:,
5471:2
5467:/
5462:Z
5458:=
5449:2
5443:N
5433:,
5430:0
5427:=
5418:1
5412:N
5402:,
5399:2
5395:/
5390:Z
5386:=
5377:0
5371:N
5344:i
5329:]
5325:)
5321:R
5317:(
5312:i
5307:P
5301:[
5297:=
5292:i
5288:x
5277:i
5254:2
5249:F
5226:)
5220:2
5215:F
5210:;
5207:M
5203:(
5197:n
5193:H
5186:]
5183:M
5180:[
5166:i
5151:)
5145:2
5140:F
5135:;
5132:M
5128:(
5122:i
5118:H
5111:)
5108:M
5105:(
5100:i
5096:w
5070:2
5065:F
5052:]
5049:N
5046:[
5043:,
5040:)
5037:N
5034:(
5027:k
5023:i
5018:w
5011:)
5008:N
5005:(
4998:1
4994:i
4989:w
4980:=
4972:]
4969:M
4966:[
4963:,
4960:)
4957:M
4954:(
4947:k
4943:i
4938:w
4931:)
4928:M
4925:(
4918:1
4914:i
4909:w
4880:n
4877:=
4872:k
4868:i
4864:+
4858:+
4853:1
4849:i
4828:1
4820:j
4816:2
4809:i
4806:,
4803:1
4797:i
4787:k
4772:)
4766:k
4762:i
4758:,
4752:,
4747:1
4743:i
4738:(
4717:,
4712:n
4706:N
4697:]
4694:N
4691:[
4688:=
4685:]
4682:M
4679:[
4669:N
4665:M
4661:n
4647:1
4639:j
4635:2
4628:i
4606:i
4602:x
4577:]
4573:1
4565:j
4561:2
4554:i
4551:,
4548:1
4542:i
4538:|
4532:i
4528:x
4523:[
4517:2
4512:F
4507:=
4496:N
4464:n
4458:N
4449:0
4446:=
4443:]
4440:M
4437:[
4427:M
4419:M
4411:M
4407:n
4391:n
4385:N
4376:]
4373:M
4370:[
4338:n
4332:N
4324:0
4318:n
4310:=
4299:N
4273:,
4270:]
4267:N
4261:M
4258:[
4255:=
4252:]
4249:N
4246:[
4243:]
4240:M
4237:[
4211:2
4206:F
4182:n
4176:N
4153:)
4150:]
4147:1
4144:,
4141:0
4138:[
4132:M
4129:(
4123:=
4120:M
4114:M
4104:M
4090:]
4084:[
4081:=
4078:]
4075:M
4072:[
4069:+
4066:]
4063:M
4060:[
4050:n
4036:]
4030:[
4008:n
4002:N
3979:]
3976:N
3970:M
3967:[
3964:=
3961:]
3958:N
3955:[
3952:+
3949:]
3946:M
3943:[
3933:N
3929:M
3909:O
3904:n
3877:n
3871:N
3858:n
3813:N
3809:W
3805:M
3797:P
3793:M
3789:W
3785:N
3780:W
3776:P
3772:N
3768:W
3764:N
3760:M
3756:W
3654:N
3650:M
3646:W
3638:f
3621:.
3616:2
3611:D
3601:1
3596:D
3584:I
3580:M
3576:W
3562:,
3559:M
3551:2
3546:D
3536:0
3531:S
3519:M
3515:N
3501:,
3496:1
3491:S
3481:1
3476:S
3471:=
3468:N
3443:2
3438:S
3433:=
3430:M
3405:3
3400:D
3390:2
3385:D
3375:1
3370:S
3365:=
3362:W
3345:f
3337:M
3333:W
3329:f
3325:M
3321:N
3317:M
3313:W
3309:f
3305:W
3301:f
3297:N
3293:f
3289:M
3285:f
3281:W
3277:f
3273:N
3269:M
3265:W
3253:N
3249:M
3245:W
3241:f
3237:W
3233:p
3229:c
3227:(
3225:f
3221:M
3217:c
3215:(
3213:f
3209:N
3205:p
3201:c
3197:n
3189:f
3159:1
3154:S
3144:1
3139:S
3113:.
3108:1
3103:D
3093:1
3088:S
3066:,
3061:2
3056:D
3046:0
3041:S
3017:2
3012:D
3002:0
2997:S
2963:2
2958:D
2948:0
2943:S
2919:1
2914:D
2904:1
2899:S
2874:.
2869:1
2864:D
2854:1
2849:S
2825:2
2820:D
2810:0
2805:S
2763:1
2758:S
2734:1
2729:S
2707:.
2702:0
2697:S
2687:1
2682:D
2658:1
2653:D
2643:0
2638:S
2581:.
2578:N
2570:1
2564:q
2559:S
2549:1
2546:+
2543:p
2538:D
2526:N
2522:M
2518:N
2514:M
2510:W
2488:)
2482:q
2477:D
2467:1
2464:+
2461:p
2456:D
2450:(
2444:}
2441:1
2438:{
2430:q
2425:D
2415:p
2410:S
2400:)
2397:I
2391:M
2388:(
2382:W
2352:.
2348:)
2342:1
2336:q
2331:S
2321:1
2318:+
2315:p
2310:D
2304:(
2297:=
2292:1
2286:q
2281:S
2271:p
2266:S
2261:=
2257:)
2251:q
2246:D
2236:p
2231:S
2225:(
2196:1
2190:q
2185:S
2175:1
2172:+
2169:p
2164:D
2140:q
2135:D
2125:p
2120:S
2090:)
2084:1
2078:q
2073:S
2063:1
2060:+
2057:p
2052:D
2046:(
2036:1
2030:q
2025:S
2015:p
2010:S
2003:|
1990:)
1980:m
1977:i
1971:t
1968:n
1965:i
1958:M
1955:(
1949:N
1936:n
1922:,
1919:M
1911:q
1906:D
1896:p
1891:S
1886::
1870:q
1866:p
1862:n
1858:M
1851:)
1849:Y
1845:X
1841:Y
1837:X
1833:Y
1829:X
1823:Y
1819:X
1797:)
1794:X
1791:(
1786:P
1783:O
1780:T
1767:,
1764:)
1761:X
1758:(
1753:L
1750:P
1689:G
1685:G
1681:G
1677:N
1673:M
1669:G
1665:W
1661:G
1638:G
1602:G
1551:N
1547:M
1483:)
1479:R
1475:(
1470:n
1467:2
1462:P
1447:n
1439:n
1435:n
1431:n
1427:M
1423:M
1415:M
1411:n
1385:M
1378:M
1356:n
1351:D
1341:0
1336:S
1310:M
1303:M
1279:M
1270:M
1250:.
1245:1
1240:S
1216:1
1211:S
1199:1
1194:S
1172:.
1165:M
1156:M
1129:M
1122:M
1112:M
1108:M
1104:n
1097:N
1093:M
1089:N
1085:M
1081:W
1074:N
1070:M
1066:N
1058:M
1043:M
1039:M
1033:M
1026:M
1020:I
1016:M
1011:M
1005:I
989:N
985:M
981:2
978:W
974:N
970:1
967:W
963:M
955:W
951:W
947:M
943:M
936:M
928:M
920:N
916:M
902:)
899:N
896:,
893:M
890:;
887:W
884:(
861:.
855:)
852:N
849:(
846:j
840:)
837:M
834:(
831:i
828:=
825:W
799:W
790:N
784:j
764:W
755:M
749:i
726:N
706:M
686:n
666:W
646:)
643:1
640:+
637:n
634:(
614:)
611:j
608:,
605:i
602:,
599:N
596:,
593:M
590:;
587:W
584:(
557:)
554:1
551:+
548:n
545:(
514:=
511:M
480:M
457:M
437:M
414:.
411:}
408:0
400:n
396:x
387:n
382:R
374:)
369:n
365:x
361:,
355:,
350:1
346:x
342:(
339:{
323:M
305:.
300:n
295:R
269:M
262:n
227:h
173:N
167:M
164:=
161:W
148:N
144:M
140:W
136:N
132:M
104:W
100:n
96:W
89:n
33:N
29:M
25:W
Text is available under the Creative Commons Attribution-ShareAlike License. Additional terms may apply.